|
|
GIZE, STONEHENGE, CHICHEN ITZA, ETC,ETC: The Keys of Atlantis-Vescica Piscis y el paralelo 30/Gran Piramide
Elegir otro panel de mensajes |
|
|

The Keys of Atlantis
A Study of Ancient Unified Numerical and Metrological Systems
by Peter Wakefield Sault
Copyright © Peter Wakefield Sault 1973-2012
All rights reserved worldwide
The Keys of Atlantis
Chapter 3.
Mountains of The Moon
The Stone-Cored Pyramids of Egypt
|
PROLEGOMENON
This little treatise is a mathematical, metrological, geographical and astronomical analysis of certain Egyptian pyramids. English translations of their ancient traditional names, less the names of the kings to whom rightly or wrongly a much later Egyptian priesthood attributed their building, are used here throughout except when quoting other authors. This is problematic since the original Great Pyramid is nowadays generally referred to as Khafre, Khephren or the Second Giza Pyramid. The original name of the pyramid now widely though erroneously known as the ‘Great Pyramid’ is Pyramid of The Horizon, or simply Horizon Pyramid. If, that is to say, ‘Horizon’ is the correct interpretation of the hieroglyph Akhet, about which some apparently justifiable doubt remains. In order to minimise the reader's possible confusion, the original and true Great Pyramid (i.e. ‘Khephren’) will here be referred to as the Grand Pyramid.
|
Sections
Illustrations
Animations
Tables
References
3-1. The Giza Complex

Figure 3-1. The Pyramids of Giza (1)

Figure 3-2. Map of The Giza Pyramid Complex
3-2. The Horizon Pyramid
The Pyramid of The Horizon, known variously although erroneously as the Pyramid of Khufu, Pyramid of Kheops, Great Pyramid or First Giza Pyramid, stands on the Giza Plateau in Egypt at 29° 58' 45" N × 31° 08' 03" E. These and all other geographical coordinates given in this chapter have been obtained since 2006 with the aid of Google Earth and supercede all earlier figures from other sources.
Peter Tompkins summarizes the origin of the Horizon Pyramid thus[1]
“Though all agree that the Great [Horizon] Pyramid is at least four thousand years old, none can say for certain just when it was built, by whom, or why.”
The first thing to note about this observation is that Tompkins refrains from attempting to assign a maximum age to the Horizon Pyramid.
Tompkins' book ‘Secrets of The Great [Horizon] Pyramid’ (1971) is without doubt the most comprehensive compendium of myths, legends and knowledge about the Horizon Pyramid ever published and was, as noted on his website*, “not yet faulted in its original content”. Until now, that is to say, firstly in respect of his misapplication of the name ‘Great’ to the Horizon Pyramid and secondly in his treatment of Isaac Newton's fictive ‘Memphis Cubit’ as if it were a genuine unit of measure when it was never more than a figment of Newton's fevered imagination. These matters will be discussed in detail in the final section of this chapter, ‘The Egyptological Fraud’.
Tompkins goes on to say[2]
“It would be satisfactory to be able to describe the method by which the Great [Horizon] Pyramid was put together, by whom, and when.
“But the builders, whoever they may have been, left no description of their method. No one has even found a later Egyptian report of how the first pyramids were built.”
The earliest extant reference to the Horizon Pyramid comes from Herodotus, who says[7]
“To build the pyramid itself took twenty years; it is square at the base, its height (800 feet) equal to the length of each side; it is of polished stone blocks beautifully fitted, none of the blocks being less than thirty feet long.”
It would be another 2,000 years before the Horizon Pyramid was accurately surveyed and the relationship between its height and the length of each side thereby properly observed. With the missing part of the statement inserted:– The quadrant whose radius is the height is equal to the length of each side.

Figure 3-3. Theoretical Proportions of the Horizon Pyramid
Some of the earlier surveys contained errors and gave rise to some preposterous theories, none of which will be discussed here, about which Prof. W.M.F.Petrie says[8]
“It is useless to state the real truth of the matter, as it has no effect on those who are subject to this type of hallucination. They can but be left with the flat earth believers and other such people to whom a theory is dearer than a fact.”
http://www.odeion.org/atlantis/chapter-3.html |
|
|
|
|
PATRON DEL NUMERO OCHO EN EL DISEÑO DE LA GRAN PIRAMIDE-BASE=2 EN EL CUADRADO DE LA PIRAMIDE IDEAL
La Gran Pirámide de Keops: pi por la raíz de fi es casi cuatro
Cuaderno de bitácora: publicamos hoy otro de los artículos que en su día aparecieron en doDK. Este artículo fue escrito hace más de seis años. En él explico un descubrimiento que hice por mí mismo, una extraordinaria coincidencia que se da en las proporciones de la Pirámide de Keops y que implica, necesariamente, una no menos extraordinaria coincidencia entre dos de los números más conocidos de las matemáticas.
[Vista de las tres grandes pirámides de la planicie de Giza o Gizeh. No hay que confundirse: la pirámide de Keops, la Gran Pirámide, es la que está más a la derecha, más atrás en la foto. La del medio es la de Kefrén, la segunda en altura, aunque en la foto parece más alta por estar más cerca, y la tercera la de más a la izquierda, la de Micerinos. La pirámide de Kefrén es muy fácil de reconocer porque conserva en su parte superior algo del revestimiento original. Es muy frecuente que se hable de la Gran Pirámide de Keops y sin embargo en las imágenes, erróneamente, aparezca la pirámide de Kefrén, la más fotogénica de las tres]
La gran pirámide de la planicie de Gizeh, la conocida como pirámide de Keops, siempre ha sido una fuente de misterios, y la mayoría están aún por resolver. Sus medidas han sido estudiadas exhaustivamente por todos los inquietos de los enigmas antiguos, y con los datos obtenidos podemos afirmar que los Egipcios no construyeron la pirámide dándole unas medidas al azar, sino que sus proporciones mantienen unas relaciones matemáticas muy interesantes entre sí.
La gran pirámide medía originalmente 147 metros de altura, y el lado de la base tenía una longitud de 230 metros, aproximadamente. Hoy en día la pirámide es un poco más baja, porque a lo largo de los siglos y sobre todo en la Edad Media ha sido utilizada de cantera artificial. Las piedras de las que estaba compuesta se han ido partiendo y tallando en ladrillos más pequeños para servir de material a algunos monumentos levantados en el pasado en la ciudad de El Cairo. Así la pirámide, que en su origen tenía una superficie pulida y blanca y estaba rematada por una punta de oro, se puede contemplar hoy como cuando contemplamos una casa vieja y a punto de derrumbarse, en la que se ven los ladrillos porque la capa de yeso que recubría la pared se ha caído con el tiempo.
Hace ya muchos años descubrí en cierto libro que las proporciones de la pirámide guardaban una importante relación: cuatro veces el lado de la base dividido por dos veces la altura daba el número pi. Esto es lo mismo que decir que si tomamos la altura de la pirámide como radio de una circunferencia, la longitud de la circunferencia coincide con el perímetro de la base.
Si tomamos como datos los que hemos mencionado anteriormente, h = 147 metros, y b = 230 metros. Haciendo la cuenta, 4·b = 920, 2·h = 294, y dividiendo ambas cantidades obtenemos 3'1292517..., es decir, aproximadamente 3'13. Teniendo en cuenta que tanto la altura de la pirámide como el lado de la base se han tomado de forma aproximada, es normal esperar que el resultado no coincida exactamente con el número pi.
Si tomamos en cuenta unas medidas más exactas, como las que aparecen en el libro De las mentiras de la Egiptología a las Verdades de la Gran Pirámide, de Luis García Gallo, la altura sería de 146'7 metros y el lado de la base de 230'4 metros (aproximadamente). Volviendo a hacer los cálculos con estas dos nuevas aproximaciones tenemos que 4·b/(2·h) = 3'14110429... y aquí ya nos vamos aproximando más al número pi. De hecho el error es del orden de 0’016%.
El error es mínimo y totalmente admisible ya que en arquitectura, lo mismo que en todas las demás ciencias aplicadas, las medidas tienen un límite de precisión. De hecho, los cuatro lados de la base de la pirámide no miden exactamente lo mismo, sino que se diferencian en algunos centímetros. De la misma forma las desaparecidas Torres Gemelas no eran exactamente igual de altas, sino que una era un poco más alta (creo que como medio metro) que la otra. A todo esto hay que añadir los estragos del tiempo sobre los monumentos. Las medidas obtenidas son aproximadas sobre una estimación de lo que la pirámide medía cuando la construyeron, hace casi cinco mil años, porque ahora las medidas son muy distintas...
La relación entre b y h se puede expresar así:
Es decir, la proporción entre b y h es como la de pi a 2.
Consultando la página de matemáticas Epsilones descubrí algo nuevo para mí. Según el historiador Heródoto, los Egipcios construyeron la gran pirámide de tal forma que el área de cada una de las caras triangulares laterales coincidiera con el área de un cuadrado de lado igual a la altura.
Teniendo en cuenta lo que acabamos de decir, nos encontramos con las siguientes fórmulas:
Vamos a buscar la proporción entre a, b y h:
Dividimos por b cuadrado y consideramos a/b como una incógnita:
Hemos suprimido la solución negativa porque tanto a como b son números positivos (estamos tratando con longitudes de la pirámide).
De repente nos ha aparecido el número áureo, fi), un número no tan conocido como pi, pero muy importante en la historia de las matemáticas:
De aquí tenemos la relación entre a y b, y por ende entre b y h:
Con esto tenemos que la proporción entre a y b es como la de fi a 2, y la proporción entre b y h es como la de 2 a la raíz cuadrada de fi.
Resumiendo, si los Egipcios construyeron la pirámide con las proporciones mencionadas por el historiador Heródoto, entonces la pirámide de Gizeh es proporcional a una que tenga como altura de una de las caras laterales a fi y como lado de la base a 2:
Entonces surge la cuestión de si ambas propiedades de la pirámide son consistentes, la de pi y la de fi. ¿Cuál de las dos propiedades es la que guió a los constructores de la pirámide? ¿O los constructores quisieron incluir adrede ambas características en su diseño?
Supongamos que somos los constructores, y el faraón nos ordena que levantemos una pirámide en la que el perímetro de la base dividido entre dos veces la altura dé el número pi. Como ya conocemos el número pi, sólo tenemos que preguntarle al faraón la altura que quiere que tenga, y tras unos cálculos sencillos, obtenemos todas las dimensiones, el lado de la base, la longitud de las aristas, etc. Pero el faraón nos dice poco después que además quiere que el área de una de las caras laterales sea igual al área de un cuadrado de lado igual a la altura.
¿Pueden ser posibles ambas cosas? Nosotros ya hemos hecho los cálculos de todas las dimensiones y ya casi nos hemos puesto manos a la obra... Sólo podemos esperar que la suerte nos acompañe y que efectivamente y casi por casualidad se cumpla la segunda condición que nos pide nuestro rey.
¡Y la suerte está de nuestro lado!
Para que se cumpla la condición de pi, b y h tienen que estar en proporción de pi a 2. Para que se cumpla la condición de fi, b y h tienen que estar en proporción de 2 a raíz de fi. Si queremos que se cumplan las dos condiciones, ambas proporciones han de ser iguales:
Bueno, esto no es cierto exactamente, pero sí aproximadamente:
De hecho el error que se comete es menor al 0'1%. Eso quiere decir que con un error del 0'1% podemos construir una pirámide que cumpla las dos condiciones, guardando dentro de sus proporciones al número pi y al número fi. Y la pirámide de Keops es un ejemplo de ello.
Maravilloso, ¿verdad? Y todo porque pi por la raíz de fi es casi cuatro.
Notas: no fui el primero en descubrir esta coincidencia entre los números pi y fi. En el libro de Martin Gardner, Los Mágicos Números del Doctor Matrix, en el capítulo de las pirámides, se habla sobre la curiosa relación entre el número pi y el número fi que posibilita que la Gran Pirámide de Keops cumpla dos propiedades matemáticas diferentes. Sin embargo, honestamente, no leí ese contenido del libro hasta este mismo año pasado, 2009, seis años después de escribir este artículo.
Por otro lado, han quedado plasmados mis esfuerzos para expresar la notación matemática en un artículo de la web. No soy muy experto todavía en esto, y la solución que encontré en su momento fue la de usar el editor matemático del Microsoft Word para escribir la expresión que quería, y luego guardar dicha expresión como archivo de imagen, para incluirlo en el artículo. Los gráficos de las pirámides los realicé con el sencillo programa Paint que viene incluido en Windows.
Para algunas otras curiosidades matemáticas de la pirámide de Keops, entre las muchas que tiene, recomiendo leer mi artículo en el blog Vientos de eternidad.
|
|
|
|
|
IMPRESIONANTE CONEXION FILIPOS (ORACULO DE DELFOS)-SO-PHI-A /1.618-ES OBVIO EN ESTE MARCO QUE NUESTRO SEÑOR HACIA REFERENCIA A MARCO ANTONIO EN MATEO 16:18-SECRETO DEL APOLO 11 DECODIFICADO-
triángulos isósceles
 |
| el templo de Athena Afaia en la isla de Aegina |
Uno del más famosa tales colocaciones es el triángulo isósceles entre el acropolis de Atenas, el templo de Poseidon en Sounion y el templo de Afaia Athena en Aegina con la distancia del estadio 242. Si era el único que podría ser justo una coincidencia pero tenemos más triángulos interesantes…
Relacionan la mayoría de ellos con Delos, Delphi y Dodoni. ¡Es otra coincidencia interesante que estos tres nombres están utilizando como primero ponen letras a la D, delta en griego, esta letra se escriben como triángulo isósceles también!

Delphi
 |
| ciudades con la misma distancia de Delphi |
El oráculo de Delphi fue creído para ser el ombligo del mundo. Pues un símbolo él recuerda a nosotros el nacimiento humano donde el ombligo pasa la vida de la madre al niño y también al punto del balance del peso que es el ombligo para el ser humano.
Aquí son algunas ciudades que tienen distancia igual del Delphi.
Como una unidad que mide nosotros utiliza el estadio = 185.2 metros.
- Atenas - Olympia (660 estadios)
- Eleusis - Iolkos (550 estadios)
- Megalopoli - Figaleia (660 estadios)
- Idaio Andro (Crete) - Smyrna (2198 estadios) - triángulo equilátero
- Pella - Corfu, (1350 estadios)
- Kinira (Thasos) - Kardamili (Hios) (1700 estadios)
- Dodoni - Dion (estadio 1010, 187 kilometerss)
 http://www.ancient-technology.com/ancient_technology_es/geometry.asp http://www.ancient-technology.com/ancient_technology_es/geometry.asp
Romanos 11:33: ¡Oh profundidad de las riquezas de la sabiduría y de la ciencia de Dios! ¡Cuán insondables son sus juicios, e inescrutables sus caminos!
FI=PHI=NUMERO DE ORO=1.618033
171. Salmos 45:9: Hijas de reyes están entre tus ilustres;Está la REINA a tu diestra con oro de Ofir.
SO-FI-A/SABIDURIA/S-OFI-A/FI-L-OFI-A
REINA VESTIDA CON ORO DE O-FI-R
triángulos isósceles
 |
| el templo de Athena Afaia en la isla de Aegina |
Uno del más famosa tales colocaciones es el triángulo isósceles entre el acropolis de Atenas, el templo de Poseidon en Sounion y el templo de Afaia Athena en Aegina con la distancia del estadio 242. Si era el único que podría ser justo una coincidencia pero tenemos más triángulos interesantes…
Relacionan la mayoría de ellos con Delos, Delphi y Dodoni. ¡Es otra coincidencia interesante que estos tres nombres están utilizando como primero ponen letras a la D, delta en griego, esta letra se escriben como triángulo isósceles también!

Delphi
 |
| ciudades con la misma distancia de Delphi |
El oráculo de Delphi fue creído para ser el ombligo del mundo. Pues un símbolo él recuerda a nosotros el nacimiento humano donde el ombligo pasa la vida de la madre al niño y también al punto del balance del peso que es el ombligo para el ser humano.
Aquí son algunas ciudades que tienen distancia igual del Delphi.
Como una unidad que mide nosotros utiliza el estadio = 185.2 metros.
- Atenas - Olympia (660 estadios)
- Eleusis - Iolkos (550 estadios)
- Megalopoli - Figaleia (660 estadios)
- Idaio Andro (Crete) - Smyrna (2198 estadios) - triángulo equilátero
- Pella - Corfu, (1350 estadios)
- Kinira (Thasos) - Kardamili (Hios) (1700 estadios)
- Dodoni - Dion (estadio 1010, 187 kilometerss)
 http://www.ancient-technology.com/ancient_technology_es/geometry.asp http://www.ancient-technology.com/ancient_technology_es/geometry.asp
|
|
FILIPOS ESTA EN EL MISMO PARALELO QUE EL VATICANO (PARALELO 41)
Filipos (ciudad)
De Wikipedia, la enciclopedia libre
Para otros usos de este término, véase Filipo. Filipos (latín Philippi, griego Φίλιπποι, Phílippï) fue una ciudad de Macedonia oriental fundada por Filipo II de Macedonia, que le dio su nombre (antes se llamaba Crénides, latín Crenides, es decir, lugar de las fuentes, por las diversas fuentes del río Angites). Cerca había minas de oro, especialmente las de Asyla. Estaba cercana al río Gangas o Gangites.
Filipos es una fundación del rey de Macedonia, Filipo II, en el lugar de la colonia tasia de Crénides (Κρηνἱδες), en el valle del monte Orbelos (nombre antiguo del monte Lekani), en el borde norte de la marisma que ocupaba en la antigüedad toda la llanura que lo separaba del monte Pangeo al sur.
El objetivo de esta fundación era tanto tomar el control de las minas de oro vecinas como establecer una guarnición en un punto de paso estratégico: el sitio controlaba la ruta entre Anfípolis y Neápolis, un segmento de la gran carretera real que atravesaba de este a oeste, Macedonia y que será más tarde reconstruida por los romanos bajo el nombre de Vía Egnatia
Filipo II dotó a la nueva ciudad de importantes fortificaciones, que cortaban en parte el paso entre las marismas y el Orbelos, y envío allí colonos. Hizo emprender el saneamiento de las marismas, de lo que da testimonio al escritor Teofrasto. Filipos conservó una verdadera autonomía dentro del Reino macedonio: una ciudad con sus propias instituciones políticas (ekklesía del demo). El descubrimiento de nuevas minas de oro cerca de la ciudad, en Asyla, contribuyó al enriquecimiento del reino de Filipo II, que estableció allí un taller monetario. La integración definitiva de la ciudad en el reino macedonio tendría lugar bajo Filipo V.
La ciudad fue a pesar de todo de tamaño modesto (¿2.000 habitantes?): cuando los romanos destruyeron definitivamente el reino argéada de Macedonia en 167 a. C. y lo dividieron en cuatro estados distintos (merides), fue Anfípolis y no Filipos la designada como capital del estado de Macedonia oriental.
No se conoce nada o casi nada de la ciudad en esta época, excepto el recinto, el teatro, los cimientos de una casa bajo el foro romano, un pequeño templo y sobre todo un heroón (templo consagrado a un héroe). Este monumento recubre la tumba de un tal Exekestos quizás situada en el ágora, y ligada al culto de κτίστης (ktístès) (héroe fundador) de la ciudad.
Hacia el 400 a. C. pasó a Tasos. Después pasó a Filipo II que explotó las minas y obtenía 1000 talentos al año. Después de la conquista macedonia de Anfípolis, Pidna y Potidea, la ciudad fue agrandada y fortificada para defenderla de las tribus tracias.

Vestigios del centro de la ciudad: foro en primer plano, mercado y basílica B al fondo.
Fue visitada en algunas ocasiones por el apóstol Pablo (49, 56 y 57). Cuando Macedonia fue dividida en dos provincias por Teodosio el Joven, Filipos fue la capital de Macedonia Primera.
La ciudad reaparece en las fuentes con ocasión de la guerra civil romana que siguió al asesinato de Julio César. Sus herederos Marco Antonio y Octavio se enfrentaron a los partidarios de la República, Marco Junio Bruto y Cayo Casio Longino, en una doble batalla decisiva en la llanura al oeste de la ciudad en octubre de 42 a. C.
Un bando estaba dirigido por Augusto y el otro por Bruto; en un primer combate este último venció, pero en el segundo, veinte días después, fue derrotado. Vencedores, Marco Antonio y Octavio licenciaron una parte de sus veteranos, probablemente de la legio XXVIII, los cuales se instalaron en la ciudad, refundada como colonia romana bajo el nombre de Colonia Victrix Philippensium.
Augusto le dio entonces, (41 a. C.) el rango de colonia (Colonia Victrix Philippensium) con derecho italiano. En 30 a. C., Octavio reorganizó la colonia y procedió a un nuevo licenciamiento de veteranos, quizás de pretorianos y de italianos: la ciudad tomó el nombre de Colonia Iulia Philippensis, convertido en Colonia Augusta Iulia Philippensis después de enero de 27 a. C., cuando Octavio recibió él mismo el nombre definitivo del Senado.

Basilíca B vista del suroeste, y acrópolis al fondo.
Pablo habría visitado la ciudad en otras dos ocasiones, en 56 y 57. La epístola a los filipenses dataría de 54-55 y testimonia el impacto inmediato de la palabra paulina. El desarrollo subsecuente del cristianismo en Filipos está bien atestiguado, sobre todo por una carta de Policarpo de Esmirna dirigida a la comunidadd filipense hacia el 160, y por la epigrafía funeraria.

Inscripción del obispo Porfirio en la Basílica de Pablo.
. El parentesco del plano y de la decoración arquitectónica de la basílica B con Santa Sofía y Santa Irene de Constantinopla otorgan un lugar privilegiado a esta iglesia en la historia del arte paleocristiano. El complejo episcopal que toma el lugar de la Basílica de Pablo a partir del siglo V, construida alrededor de una iglesia octogonal, rivaliza también con las iglesias de la capital.
MARY MAGDALENE (ESTRELLA DE OCHO PUNTAS)-OCTAVO DIA
En la misma época, las murallas de la ciudad son reconstruidas para hacer frente a la inseguridad creciente en los Balcanes. ·n 473, la ciudad es sitiada por los ostrogodos que no llegan a tomarla pero queman los arrabales.
|
|
|
|
|
La conexion MATEO 16;18 esta en el contexto a LA BATALLA DE FILIPO Y MARCO ANTONIO. EL ARCA DE NOE TAMBIEN ESTA DISEÑADA CON EL PATRON DE LA SERIE DE FIBONACCI. ESTO EXPLICA DESDE OTRA PERSPECTIVA LA RELACION COLUMBIA CON EL APOLO 11 (ORACULO DE DELFOS).
|
|
|
|
|
|
|
171. Salmos 45:9: Hijas de reyes están entre tus ilustres;Está la REINA a tu diestra con oro de Ofir.
SO-FI-A/SABIDURIA/S-OFI-A/FI-LOS-OFI-A
REINA VESTIDA CON ORO DE O-FI-R
OFIR/OPHIR
ETIOPIA
BETH-SABE (CASA DE SABA) (MADRE DEL REY SALOMON)
SABADO/SABIDURIA/REINA DE SABA
OFIDIOS
Serpentes
De Wikipedia, la enciclopedia libre
Para los municipios español y cubano, véase La Sierpe.
Las serpientes (Serpentes) u ofidios (Ophidia)/ETIOPIA/REINA DE SABA/ SABIDURIA / SOPHIA/ SABADO / SHABBAT son un suborden de saurópsidos (reptiles) diápsidos pertenecientes al orden Squamata, superorden de los Lepidosaurios, caracterizado por la ausencia de patas (la pitón mantiene diminutas extremidades fruto de su proceso evolutivo)[1] y el cuerpo muy alargado. Se originaron en el período Cretácico.
Las serpientes se caracterizan por la ausencia de extremidades y cuerpo alargado. Algunas poseen mordeduras venenosas, como las cobras y las víboras, que utilizan para matar a sus presas antes de ingerirlas. Otras serpientes, como las boas y pitones, matan a sus presas por constricción.
Se reconocen más de 450 géneros y de 3400 especies.[2] [3] Evidentemente, las serpientes derivan de algún tipo de lagarto, pero los detalles concretos de su origen no están claros.[4]

Anatomía de una serpiente.1 esófago, 2 tráquea, 3 pulmones traqueales, 4 pulmón izquierdo rudimentario, 5 pulmón derecho, 6 corazón, 7 hígado, 8 estómago, 9 saco de aire, 10 vesícula biliar, 11 páncreas, 12 bazo, 13 intestino, 14 testículos, 15 riñones.
La piel de la serpiente está recubierta por escamas. La mayoría de las serpientes utilizan escamas de la parte ventral para desplazarse, aferrándose con ellas a las superficies. Sus párpados se encuentran permanentemente cerrados, pero son en realidad escamas transparentes. Las serpientes cambian de piel periódicamente. A diferencia de otros reptiles, el cambio de piel es realizado en una pieza, como si tirara de una media. Esto se hace a medida que el animal crece y además para reparar heridas y librarse de parásitos externos. La renovación periódica ha convertido a la serpiente en un símbolo de salud y medicina, como se muestra en la vara de Esculapio
La locomoción se realiza mediante ondulaciones laterales del cuerpo; las escamas ventrales ensanchadas de muchas serpientes impiden el deslizamiento en dirección contraria a la marcha. Algunas serpientes, como ciertas boas y las víboras pueden desplazarse también mediante movimientos musculares de las escamas ventrales con su cuerpo extendido casi en línea recta.
El esqueleto tiene muchas vértebras, 200 en las víboras y 400 en las pitones.
El cráneo está muy modificado respecto al modelo básico diápsido. Los huesos de la mandíbula superior están débilmente unidos al resto del cráneo y el cuadrado puede moverse libremente y posee potentes músculos refractores, lo que permite una enorme abertura de las mandíbulas y la deglución de grandes presas enteras. Los dientes son agudos y curvados hacia atrás y se implantan tanto en el paladar como en el maxilar y el dentario.
Las boas y las pitones poseen rudimentos de las extremidades posteriores y de sus cinturas; en estas serpientes pueden verse unas garras en la parte externa y a cada lado de la cloaca que desempeñan un cierto papel en el coito.
Existen varios tipos de dientes, según el hueso sobre el que se implanten: maxilares, pterigoideos, palatinos, dentarios y premaxilares. Los dientes maxilares son los más variados y los únicos que pueden estar asociados a glándulas venenosas; se pueden distinguir cuatro tipos principales:
Son dientes macizos, prensiles, curvados hacia atrás para sujetar la presa y no están diseñados para inocular veneno. Es el caso de muchos colúbridos, boidos y pitónidos. En general son serpientes inofensivas para el hombre, con excepción de las grandes constrictoras (pitones, anacondas).
Son dientes acanalados situados en la parte posterior de la mandíbula y conectados con glándulas de veneno, constituyendo un sistema de inoculación primitivo. Dado que para inyectar el veneno debe morder con la parte posterior de la boca, normalmente son poco peligrosas para el hombre. Este es el caso de la culebra bastarda (Malpolon monspessulanus). No obstante, las especies de gran tamaño pueden producir graves mordeduras e incluso la muerte, como Dispholidus typus, del África subsahariana.
Son dientes pequeños y fijos situados en la parte delantera de la boca, con un canal más o menos cerrado. Las cobras y las mambas poseen estos dientes. Algunas especies, como la cobra escupidora (Naja nigricollis), los tienen modificados para escupir el veneno a más de cuatro metros de distancia.[5]
Se trata de dos largos colmillos móviles en parte anterior de la mandíbula; son huecos con un canal interior cerrado y conectado con glándulas venenosas. Los colmillos se pliegan sobre el paladar superior cuando el animal cierra la boca y se enderezan rápidamente cuando la abre. Es el sistema de inoculación más eficaz. Este tipo de dentición es característico de los vipéridos.
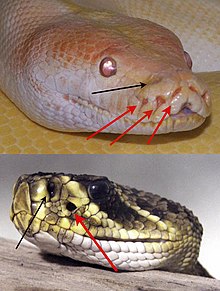
Flechas rojas: órganos receptores de infrarrojos; flechas negras: orificios nasales. Arriba, una pitón; abajo, una serpiente de cascabel
|
|
|
|
|
|
PART 4 - THE GREAT PYRAMID AND THE 30th PARALLEL
|
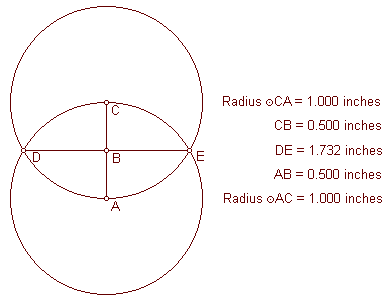
This circle has a different orientation than the previous diagrams and is two inches in diameter.
The horizontal axis is the Equator, FC is the 30th parallel, D is 60� North latitude and E is the North Pole.
The 30th parallel is exactly one-third of the great circle distance from the Equator to the North Pole, and it is located at exactly one-half of the height of the Northern Hemisphere.
Like the Great Pyramid, the maximum latitude of the line of ancient sites is very close to the 30th parallel.
|

|
|
|
This diagram illustrates that the relationship of the 30th parallel to the circumference of the Earth is the geometric relationship known as the Vesica Pisces.
In relation to the lower circumference, DE is at 30� N latitude.
In relation to the upper circumference, DE is at 30� S latitude.
The ratio between the straight line distance of the 30th parallel and the radius of the Earth is 1.732 to one. 1.732 is the square root of three.
|
|
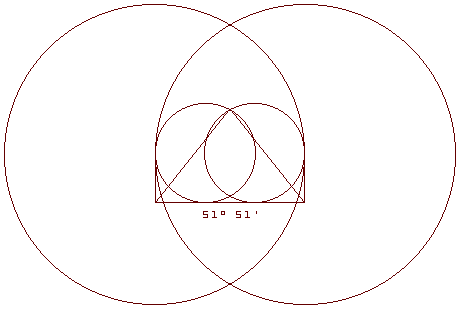
Paul Michell and Charles Henry have noted the relationship between the Great Pyramid and the Vesica Pisces.
The small circles in this diagram are one inch in diameter, and the large circles are three inches in diameter, forming a small Vesica Pisces circumscribed by a larger one.
The triangle in this diagram has the same angular dimensions as the Great Pyramid.
|
|
|
|
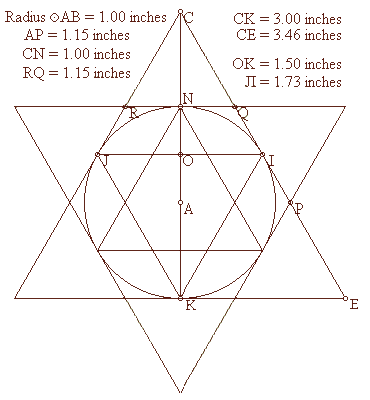
The circle in this diagram also represents the circumference of the Earth with the poles on the vertical axis.
The radius of the circle is 1.00 inch.
The exterior and interior equilateral triangles touch the circle only at the 30th parallels and the poles.
The height of each equilateral triangle is 87% of the length of each of it’s sides:
3.00 � 3.46 = .87
1.50 � 1.73 = .87
1.00 � 1.15 = .87
The length of each of the sides of the interior triangles, including the straight line distance through the earth at the 30th parallel, is also 87% of the diameter of the Earth:
1.73 � 2.00 = .87
|
The radius of the Earth is also 87% of the distance from the center of the Earth to the point of the exterior triangles’ intersections (AP, AQ, AR, etc.):
1.00 � 1.15 = .87
The Greek foot is thought to have been developed before the size and shape of the Earth was known and independently from the foot, which is also thought to have been developed before the size and shape of the Earth was known. The foot is 87% of the length of the Greek foot.
The mile, which is thought to have been developed before the size and shape of the Earth was known, is 87% of the length of the nautical mile, which was developed specifically in relation to the size of the Earth. The nautical mile equals one minute of latitude, so 60 nautical miles equals one degree of latitude and 5,400 nautical miles equals the 90 degrees of latitude between the Equator and the poles.
The distance from the Equator to the poles is 6,215 miles:
5,400 � 6,215 = .87
One minute of latitude equals one nautical mile at any longitude.
At the equator, one minute of longitude also equals one nautical mile, but at higher latitudes, the distances between each minute of longitude become shorter. Because the straight line distance through the Earth at the 30th parallel is 87% of the diameter of the Earth, the circumference around the Earth at the 30th parallel is 87% of the circumference of the Earth at the Equator, and each minute of longitude at the 30th parallel is 87% of the distance of each minute of longitude at the Equator. As a result, just as one nautical mile equals one minute of longitude at the Equator, one standard mile equals one minute of longitude at the 30th parallel.
The currently accepted value for the Equatorial diameter of the Earth is 7,926 miles, with an Equatorial radius of 3,963 miles. The ratio of the radius of the Earth to the straight line distance through the Earth at the 30th parallel is 1:1.732.
3,963 miles x 1.732 = 6,864 miles (the straight line distance through the Earth at the 30th parallel).
6,864 miles x pi = 21,564 miles (the circumference of the Earth at the 30th parallel).
21,564 miles � 360 degrees = 59.9 miles (one degree of longitude at the 30th parallel).
59.9 miles � 60 minutes = .998 miles (one minute of longitude at the 30th parallel).
As an alternative proof, the currently accepted value for the Equatorial circumference of the Earth is 24,902 miles, and the circumference of the 30th parallel is 87% of the circumference of the Earth.
24,902 miles x .87 = 21,664 miles (the circumference of the Earth at the 30th parallel).
21,664 miles � 360 degrees = 60.1 miles (one degree of longitude at the 30th parallel).
60.1 miles � 60 = 1.00 miles (one minute of longitude at the 30th parallel).
Conclusion: One minute of longitude equals one mile at (or, like the Great Pyramid, just below) the 30th parallel.
Back to Contents
|
|
|
|
|
|
|
En el contexto a lo anteriormente expresado, CRISTO HABRIA NACIDO EL 25 DE DICIEMBRE DEL AÑO 13 A.C, ya que en ese año se CUMPLIO EL PATRON LUNAR DE LUNA LLENA EL 19 DE MARZO. OSEA QUE CRISTO HABRIA SIDO CRUCIFICADO A SUS 44 AÑOS Y 81 DIAS, ya que en el año 32 D.C cumplio esa cantidad de años. OSEA QUE EL AÑO JULIANO-GREGORIANO esta en funcion a SU 12 ANIVERSARIO DE CIRCUNCISION, QUE INSISTO FUE EN EL MISMO AÑO NUEVO. ESE SERIA EL MENSAJE, EN EL CONTEXTO AL EVANGELIO, DE SU PRESENTACION EN EL TEMPLO EN EL CONTEXTO A LA PASCUA CUANDO TENIA 12 AÑOS. TODO ESTO, OBVIAMENTE EN EL CONTEXTO A QUE SI TENEMOS REFERENCIA A QUE LA LUNA NUEVA ES EN LUNA LLENA. INSISTO, QUE ES GRANDE ESTA PROBABILIDAD, EN EL CONTEXTO A QUE SU CRUCIFICCION FUE EN EL 15 DE MARZO (MISMO DIA DEL ASESINATO DEL CESAR), QUE TAMBIEN ES UNA REFERENCIA A VESICA PISCIS (153 PECES= JUAN 21:11) EN EL CONTEXTO A LA LANZA DE LONGINO. TODO ESTO CODIFICADO EN LA MISMA MISA CATOLICA. RECORDEMOS TAMBIEN QUE VENUS, QUE TIENE FUERTE RELACION CON JACOB, TIENE UN CICLO DE 40 AÑOS SOLARES=8*5 AÑOS SOLARES, EN EL CONTEXTO A QUE EN 8 AÑOS SOLARES TENEMOS 13 PERIODOS SIDEREOS DE VENUS. EL SACRIFICIO DE CRISTO TIENE FUERTE NEXO CON DICHO PLANETA, EN EL CONTEXTO A JACOB=VENCEDOR = VENUS. EN REALIDAD EL NUMERO 8= INFINITO= RESURRECCION = "VIDA ETERNA", EN EL CONTEXTO AL NUMERO 13 (SANTA CENA) , INSISTO TIENE FUERTE NEXO CON VENUS (SERIE DE FIBONACCI= NUMERO DE ORO PHI= 1.618033). ESTO ES ALQUIMIA PURA. JESUCRISTO ES EL LUCERO DE LA MAÑANA, OSEA VENUS EN EL MISMO CONTEXTO A APOCALIPSIS 22:16. EL PIE DE JACOB (COJEABA), ES UNA REFERENCIA AL MISMO PIE DE OPHIUCO. CATOLICOS, ACONDEJO IR A MISA Y ESTUDIAR EL ESOTERISMO BIBLICO. EL SACRIFICIO DE LA SANTA MISA, ESTA EN FUNCION A VESICA PISCIS, OSEA LA "MAQUINA DEL TIEMPO".
|
|
|
|
|
|
|
| Reply |
Message 24 of 24 on the subject |
|
|
| Reply |
Message 172 of 172 on the subject |
|
|
|
|
|
|
|
|
|
|
|
|
|
La razon de la circunferencia y el diametro es obvio que es el numero PI.
|
|
|
|
|
|
22/7=DIA DE MARIA LA MAGDALENA=3.14 =PI
|
|
|
| Reply |
Message 61 of 63 on the subject |
|
|
|
|
| Reply |
Message 62 of 63 on the subject |
|
|
|
|
| Reply |
Message 63 of 63 on the subject |
|
|
|
|

SABIDURIA=SABADO=LETRA S=$
|
|
|
|
|
|
y la Omega, principio y fin, dice el Señor, el que es y que era y que ha de venir, el Todopoderoso.
16. Apocalipsis 1:11 que decía: Yo soy el ALFA y la Omega, el primero y el último. Escribe en un libro lo que ves, y envíalo a las siete iglesias que están en Asia: a Efeso, Esmirna, Pérgamo, Tiatira, Sardis, Filadelfia y Laodicea.
17. Apocalipsis 2:27 y las regirá con vara de hierro, y serán quebradas como vaso de ALFArero; como yo también la he recibido de mi Padre;
18. Apocalipsis 21:6 Y me dijo: Hecho está. Yo soy el ALFA y la Omega, el principio y el fin. Al que tuviere sed, yo le daré gratuitamente de la fuente del agua de la vida.
19. Apocalipsis 22:13 Yo soy el ALFA y la Omega, el principio y el fin, el primero y el último.
|
|
|
|
|
|
|
|
|
|
|
| Alcoseri (Original message) |
Sent: 21/09/2016 02:11 |
LA CLAVE DEL EXPERIMENTO FILADELFIA
SAR (PRINCIPE)= LETRA S= SABIDURIA (SABADO)= SERPIENTE= $= RIO NILO (FORMA DE SERPIENTE)= RIO TIBER (FORMA DE SERPIENTE)
|
|
|
| Reply |
Message 5 of 7 on the subject |
|
32. Génesis 30:6 Dijo entonces Raquel: Me juzgó Dios, y también oyó mi voz, y me dio un hijo. Por tanto llamó su nombre DAN.

- Mateo 6:33: Mas buscad primeramente el reino de Dios y su JUSTICIA, y todas estas cosas os serán añadidas.
|
|
|
| Reply |
Message 6 of 7 on the subject |
|
|
|
|
| Reply |
Message 7 of 7 on the subject |
|
|
| Reply |
Message 85 of 85 on the subject |
|
|
|
|
|
|
|
|
|
|
|
| rom: Cajeli (Original message) |
Sent: 03/09/2017 20:30 |
|
LA TORRE DE MAGDALA
De María Magdalena se cree que su nombre indica que ella pertenecía a la ciudad de Magdala, del hebreo migdál que significa “ciudad de las torres”, la cual ha querido ser asociada con un puerto pesquero, aledaño a una región donde proliferaban los burdeles.
Su fama de prostituta se origina cuando la iglesia católica la quiso identificar con la mujer pecadora que ungió con perfumes a Jesús, en la casa de Simón el fariseo; sin embargo el pasaje de Lucas 7.36-50 donde se registra este hecho, no da el nombre de la mujer a quien Jesús le perdona sus pecados, ni mencionan los 7 demonios de los que Jesús libró a la Magdalena.
Aunque en el Evangelio no se dice que ella fuera viuda o casada, tampoco se dice que fuera soltera, o una prostituta. Estuvo relacionada con mujeres importantes como la esposa del intendente de Herodes, y ayudó a financiar con sus bienes el ministerio de Jesús. Teniendo en cuenta que migdal significa torre, el nombre de María Magdalena la muestra como mujer vigilante, como una atalaya.
Precisamente esta mujer permaneció atenta a todos los acontecimientos durante el ministerio de Jesús; estuvo presente “mirando de lejos” la crucifixión del Señor, junto con el grupo de mujeres que lideraba; fue ella quien vio el lugar donde Jesús era puesto luego de morir. También fue quien vio quitada la piedra del sepulcro el primer día de la semana; y fue quien primero vio a Jesús resucitado, y salió apresurada para anunciar las Buenas Nuevas.
Con su don especial de permanecer atenta, se asemeja a las cinco vírgenes sensatas que manutuvieron aprovisionadas sus lámparas hasta la llegada del Novio. La bendigo, y reconozco que la iglesia de Jesucristo necesita Atalayas como esta mujer, la cual representa a la iglesia fiel y vigilante que será la esposa del Cordero

|
 First First  Previous 2 to 12 of 12 Next Previous 2 to 12 of 12 Next  Last Last  |
| Reply |
Message 2 of 12 on the subject |
|
| From: Rolmen |
Sent: 03/09/2017 22:32 |
|
Muy bonito cuento, ¿Quién es el autor? Quizá Wilde, o tal vez Andersen, o Dumas? O sería el judío Raigorodsky? Qué sé yo, pero el cuento es fascinante, sobretodo, en la parte narrativa en la cual especifica que estuvo, María Magdalena, presente en muchos acontecimientos y me pregunto, de que viviría esa mujer que tenía espacio de tiempo para estar de fisgona, de verdad que se las inventan para tener entretenidos a los lectores creyentes de fábulas y la iglesia católica no pierde tiempo, la declara santa para seguir agregando adeptos y claro, digo esto esperando no ofender a nadie.
|
|
|
| Reply |
Message 3 of 12 on the subject |
|
| From: Cajeli |
Sent: 03/09/2017 22:43 |
|
La historia en realidad es algo más profunda.
María Magdalena era una mujer muy joven que cuidaban sus padres sabiendo que estaba endemoniada.
Sufría de neurosis y epilepsia, de esa manera se reflejaba su enfermedad espiritual, y por esto fue sanada por Jesús. No hay bases para afirmar que era prostituta, eso fue una enseñanza falsa, o mal entendido desde que en un sermón al Papa Gregorio I se le ocurrió decir que fue la mujer pecadora que ungió a Jesús con perfumes
|
|
|
| Reply |
Message 4 of 12 on the subject |
|
| From: Rolmen |
Sent: 04/09/2017 00:26 |
|
Bueno Cajeli, real o no, es irrelevante, pero si, creo es una fantasía, es como tú mismo afirmas que el tal Gregorio I se le ocurrió que dicho personaje era una trabajadora sexual, bien pudo este sujeto u otro crear o tergiversar historias que luego, repetidas una y mil veces, con el tiempo se dan como verídicas, hay quienes afirman que esta mujer fue amante de Jesús Cristo e incluso tuvo vástagos de esa relación y no lo afirmo yo, sino está en boca de mucha gente y por ello te digo que las historias están trabucadas.
|
|
|
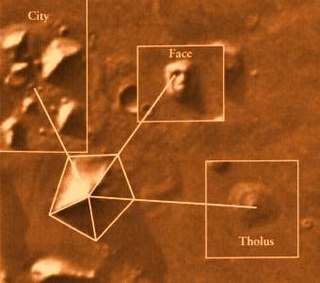
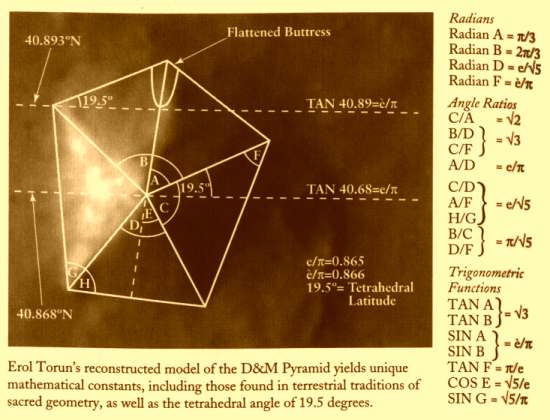
Esta es otra región de Marte, Cydonia Mensae a 33ºN y 13ºW

sidon esta en el paralelo 33
CYDONIA EN MARTE Y EL 33
| Reply |
Message 5 of 12 on the subject |
|
| From: Cajeli |
Sent: 04/09/2017 00:32 |
|
Este pasaje puede relacionarse con María Magdalena...
MATEO
15:21 Saliendo Jesús de allí, se FUE A LA REGIÓN DE TIRO Y SIDÓN.
15:22 Y he aquí una mujer cananea que había salido de aquella región clamaba, diciéndole: ¡Señor, Hijo de David, ten misericordia de mí! Mi hija es gravemente atormentada por un demonio.
15:23 Pero Jesús no le respondió palabra. Entonces acercándose sus discípulos, le rogaron, diciendo: Despídela, pues da voces tras nosotros.
15:24 El respondiendo, dijo: No soy enviado sino a las ovejas perdidas de la casa de Israel.
15:25 Entonces ella vino y se postró ante él, diciendo: ¡Señor, socórreme!
15:26 Respondiendo él, dijo: No está bien tomar el pan de los hijos, y echarlo a los perrillos.
15:27 Y ella dijo: Sí, Señor; pero aun los perrillos comen de las migajas que caen de la mesa de sus amos.
15:28 Entonces respondiendo Jesús, dijo: Oh mujer, grande es tu fe; hágase contigo como quieres. Y su hija fue sanada desde aquella hora.
15:29 Pasó Jesús de allí y vino junto al mar de Galilea; y subiendo al monte, se sentó allí.
15:30 Y se le acercó mucha gente que traía consigo a cojos, ciegos, mudos, mancos, y otros muchos enfermos; y los pusieron a los pies de Jesús, y los sanó;
15:31 de manera que la multitud se maravillaba, viendo a los mudos hablar, a los mancos sanados, a los cojos andar, y a los ciegos ver; y glorificaban al Dios de Israel.
15:32 Y Jesús, llamando a sus discípulos, dijo: Tengo compasión de la gente, porque ya hace tres días que están conmigo, y no tienen qué comer; y enviarlos en ayunas no quiero, no sea que desmayen en el camino.
15:33 Entonces sus discípulos le dijeron: ¿De dónde tenemos nosotros tantos panes en el desierto, para saciar a una multitud tan grande?
15:34 Jesús les dijo: ¿Cuántos panes tenéis? Y ellos dijeron: Siete, y unos pocos pececillos.
15:35 Y mandó a la multitud que se recostase en tierra.
15:36 Y tomando los siete panes y los peces, dio gracias, los partió y dio a sus discípulos, y los discípulos a la multitud.
15:37 Y comieron todos, y se saciaron; y recogieron lo que sobró de los pedazos, siete canastas llenas.
15:38 Y eran los que habían comido, cuatro mil hombres, sin contar las mujeres y los niños.
15:39 Entonces, despedida la gente, entró en la barca, y vino a la región de Magdala.
SIRIO ES LA CONSTELACION DEL PERRO/ CAN MAYOR
NOTEN EL NEXO DE DAN, CON LA SERPIENTE (VENECIA) Y EL CABALLO (PLAZA SAN MARCOS)
7. Génesis 49:17 Será Dan SERPIENTE junto al camino, Víbora junto a la senda, Que muerde los talones del caballo, Y hace caer hacia atrás al jinete.
VENECIA=PLAZA SAN MARCOS
11. Proverbios 25:11 MANZANA de oro con figuras de plata Es la palabra dicha como conviene.
12. Cantares 2:5 Sustentadme con pasas, confortadme con MANZANAs; Porque estoy enferma de amor.
13. Cantares 7:8 Yo dije: Subiré a la palmera, Asiré sus ramas. Deja que tus pechos sean como racimos de vid, Y el olor de tu boca como de MANZANAs,
ALLI ESTA LA CONEXION VENUS / VENECIA CON EL 666
VENUS= PENTAGONO= VENECIA= NUMERO DE ORO= 1.618033
LAS MATEMATICAS SON EXACTAS.
GLORIA A DIOS TODOPODEROSO. QUE BELLAS SON LAS MATEMATICAS
|
|
|
|
|
|
|



SIRIO ES LA CONSTELACION DEL PERRO/ CAN MAYOR
 EL MISMO DISEÑO DE ORION EL MISMO DISEÑO DE ORION
99. Ezequiel 22:7: Al padre y a la madre despreciaron en ti; al EXTRANJERO trataron con violencia en medio de ti; al huérfano y a la viuda despojaron en ti.
HECHOS 12:12 (MARCOS/ MARTE / MARZO/ PASCUA)
NOTEN EL NEXO DE DAN, CON LA SERPIENTE (VENECIA) Y EL CABALLO (PLAZA SAN MARCOS)
7. Génesis 49:17 Será Dan SERPIENTE junto al camino, Víbora junto a la senda, Que muerde los talones del caballo, Y hace caer hacia atrás al jinete.
VENECIA=PLAZA SAN MARCOS
11. Proverbios 25:11 MANZANA de oro con figuras de plata Es la palabra dicha como conviene.
12. Cantares 2:5 Sustentadme con pasas, confortadme con MANZANAs; Porque estoy enferma de amor.
13. Cantares 7:8 Yo dije: Subiré a la palmera, Asiré sus ramas. Deja que tus pechos sean como racimos de vid, Y el olor de tu boca como de MANZANAs,
ALLI ESTA LA CONEXION VENUS / VENECIA CON EL 666
VENUS= PENTAGONO= VENECIA= NUMERO DE ORO= 1.618033
LAS MATEMATICAS SON EXACTAS.
GLORIA A DIOS TODOPODEROSO. QUE BELLAS SON LAS MATEMATICAS
|
|
|
|
|
 the Apple 
|
|
|
|
|
LLAVE DE ORO Y DE PLATA AL IGUAL QUE LA MANZANA
|
|
|
|
|
22/7=3.14=PI
12 PUNTOS EN SU CIRCUNFERENCIA=RELOJ=SANTA CENA
LONGITUD CIRCUNFERENCIA=PIxDIAMETRO
 |
|
|
|
|
|
|
|
|
|
|
ISLA SAN GIORGIO (VENECIA)=GEORGE LEMAITRE
GEMATRIA EN INGLES DE SEED=33
GEMATRIA EN INGLES DE GATE=33
SARA (CE-SAREA DE FILIPO)=PARALELO 33
 the Apple
| milky way in Simple Gematria Equals: 119 |
( |
m 13 |
i9 |
l 12 |
k 11 |
y 25 |
0 |
w 23 |
a1 |
y 25 |
) |
| queen mary in Simple Gematria Equals: 119 |
( |
q 17 |
u 21 |
e5 |
e5 |
n 14 |
0 |
m 13 |
a1 |
r 18 |
y 25 |
|
| hebrew calendar in Simple Gematria Equals: 119 |
( |
h8 |
e5 |
b2 |
r 18 |
e5 |
w 23 |
0 |
c3 |
a1 |
l 12 |
e5 |
n 14 |
d4 |
a1 |
r 18 |
| mary magdalene in Simple Gematria Equals: 119 |
( |
m 13 |
a1 |
r 18 |
y 25 |
0 |
m 13 |
a1 |
g7 |
d4 |
a1 |
l 12 |
e5 |
n 14 |
e5 |
|

|
|
|
|
|
Iglesia de Santa María Madalena, Isla do Pico
Admira la Iglesia de Santa María Madalena en Madalena, Isla do Pico, Azores.
 Iglesia de Santa María Madalena © Carlos Luis M C da Cruz La Iglesia de Santa María Madalena se encuentra localizada en el casco histórico de Madalena, la capital de la Isla do Pico del archipiélago de las Azores, Portugal.
Se trata de un templo del siglo XVII, el más grande de la isla. Cuenta con dos hermosas torres gemelas coronadas por agujas de base hexagonal y decorativa peineta con un reloj del siglo XIX.
Su interior barroco presenta una serie de azulejos historiados, imágenes religiosas, y dos altares laterales y una capilla mayor con una tallas doradas
Más información:
Ubicación:
Largo Cardenal Costa Nunes, Madalena, Isla do Pico, Azores.
|
|
|
|
|
|
De: khanjee |
Enviado: 17/10/2025 15:00 |
Can I say such a relief to discover someone that actually knows what theyre referring to over the internet. You definitely understand how to bring a concern to light and work out it crucial. More people need to see this and can see this side of the story. I cant think youre less well-known as you definitely contain the gift. Patrick Market |
|
|
 Primer Primer
 Anterior
50 a 64 de 64
Siguiente Anterior
50 a 64 de 64
Siguiente
 Último
Último
|
|
| |
|
|
©2025 - Gabitos - Todos los derechos reservados | |
|
|

