|
جواب |
رسائل 1 من 166 في الفقرة |
|
http://www.youtube.com/watch?v=j9e0auhmxnc
Phi in the Bible
Although perhaps not immediately obvious, phi and the golden section also appear in the Bible. Also see the Theology page.
The Ark of the Covenant is a Golden Rectangle
|

|
In Exodus 25:10, God commands Moses to build the Ark of the Covenant, in which to hold His Covenant with the Israelites, the Ten Commandments, saying,
"Have them make a chest of acacia wood-
two and a half cubits long,
a cubit and a half wide,
and a cubit and a half high."
|
The ratio of 2.5 to 1.5 is 1.666..., which is as close to phi (1.618 ...) as you can come with such simple numbers and is certainly not visibly different to the eye. The Ark of the Covenant is thus constructed using the Golden Section, or Divine Proportion. This ratio is also the same as 5 to 3, numbers from the Fibonacci series.
In Exodus 27:1-2, we find that the altar God commands Moses to build is based on a variation of the same 5 by 3 theme:
"Build an altar of acacia wood, three cubits high; it is to be square, five cubits long and five cubits wide."
Note: A cubit is the measure of the forearm below the elbow.
|
 |
|
Noah's Ark uses a Golden Rectangle
|
In Genesis 6:15, God commands Noah to build an ark saying,
"And this is the fashion which thou shalt make it of: The length of the ark shall be three hundred cubits, the breadth of it fifty cubits, and the height of it thirty cubits."
Thus the end of the ark, at 50 by 30 cubits, is also in the ratio of 5 to 3, or 1.666..., again a close approximation of phi not visibly different to the naked eye. Noah's ark was built in the same proportion as ten arks of the covenant placed side by side.
|

|
The Number 666 is related to Phi
|

|
Revelation 13:18 says the following:
"This calls for wisdom. If anyone has insight, let him calculate the number of the beast, for it is a man's number. His number is 666."
|
| This beast, regarded by some as the Anti-Christ described by John, is thus related to the number 666, one of the greatest mysteries of the Bible.
Curiously enough, if you take the sine of 666º, you get -0.80901699, which is one-half of negative phi, or perhaps what one might call the "anti-phi." You can also get -0.80901699 by taking the cosine of 216º, and 216 is 6 x 6 x 6.
The trigonometric relationship of sine 666º to phi is based on an isosceles triangle with a base of phi and sides of 1. When this triangle is enclosed in a circle with a radius of 1, we see that the lower line, which has an angle of 306º on the first rotation and 666º on the second rotation, has a sine equal to one-half negative phi.
In this we see the unity of phi divided into positive and negative, analogous perhaps to light and darkness or good and evil. Could this "sine" be a "sign" as well?
In addition, 666 degrees is 54 degrees short of the complete second circle and when dividing the 360 degrees of a circle by 54 degrees you get 6.66... The other side of a 54 degree angle in a right angle is 36 degrees and 36 divided by 54 is .666.
Phi appears throughout creation, and in every physical proportion of the human body. In that sense it is the number of mankind, as the mysterious passage of Revelation perhaps reveals.
Also see the Theology page.
The colors of the Tabernacle are based on a phi relationship
The PhiBar program produces the colors that the Bible says God gave to Moses for the construction of the Tabernacle.
As it says in Exodus 26:1, "Make the tabernacle with ten curtains of finely twisted linen and blue, purple and scarlet yarn, with cherubim worked into them by a skilled craftsman."
Set the primary color of the PhiBar program to blue, the secondary color of the PhiBar to purple and it reveals the Phi color to be scarlet.
This reference to the combination blue, purple and scarlet in the construction of the tabernacle appears 24 times in Exodus 25 through 39, describing the colors to be used in the curtains, waistbands, breastpieces, sashes and garments.
See the Color page for additional information.
|
Insights on the Ark of the Covenant and 666 contributed by Robert Bartlett.
Insights on the Altar in Exodus 27 contributed by Sir Hemlock.
Insights on the Tabernacle colors contributed by J.D. Ahmanson.
http://goldennumber.net/bible.htm
|
|
|
|
جواب |
رسائل 32 من 166 في الفقرة |
|
God's Handiwork -- Fibonacci Numbers
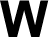 hat are Fibonacci numbers? Discovered by Leonardo Fibonacci (1170-1250 A.D.) who was born in Pisa, Italy, the Fibonacci sequence is an infinite sequence of numbers, beginning: 1, 1, 2, 3, 5, 8, 13, 21, 34, 55, 89, 144, ... where each number is the sum of the two preceding it. Thus: 1+1=2, 1+2=3, 2+3=5, 3+5=8, 5+8=13 and so on. For any value larger than 3 in the sequence, the ratio between any two consecutive numbers is 1:1.618, or the Golden Ratio. hat are Fibonacci numbers? Discovered by Leonardo Fibonacci (1170-1250 A.D.) who was born in Pisa, Italy, the Fibonacci sequence is an infinite sequence of numbers, beginning: 1, 1, 2, 3, 5, 8, 13, 21, 34, 55, 89, 144, ... where each number is the sum of the two preceding it. Thus: 1+1=2, 1+2=3, 2+3=5, 3+5=8, 5+8=13 and so on. For any value larger than 3 in the sequence, the ratio between any two consecutive numbers is 1:1.618, or the Golden Ratio.
Known by the Greek letter phi, the Golden Ratio is an irrational number (i.e. one that cannot be expressed as the ratio or fraction of two whole numbers) with several curious properties. We can define it as the number that is equal to its own reciprocal plus one: phi = 1/phi + 1, with its value commonly expressed as 1.618,033,989. It's digits were calculated to ten million places in 1996, and they never repeat. It is related to Fibonacci numbers in that if you divide two consecutive numbers in the Fibonacci sequence, the answer is always an approximation of phi.
Also known as the Divine Proportion, the Golden Mean, or the Golden Section, this ratio is found with surprising frequency in natural structures as well as man-made art and architecture. where the ratio of length to width of approximately 1:1.618 is seen as visually pleasing. It is the point dividing a distance into two segments where the proportion of the smaller segment to the larger segment is the same as the larger to the whole.
Leaves On A Stem
If we look down on a plant, the leaves are often arranged so that leaves above do not hide leaves below. This means that each gets a good share of the sunlight and catches the most rain to channel down to the roots as it runs down the leaf to the stem.
The Fibonacci numbers occur when counting both the number of times we go around the stem, going from leaf to leaf, as well as counting the leaves we meet until we encounter a leaf directly above the starting one. If we count in the other direction, we get a different number of turns for the same number of leaves. One estimate is that 90 percent of all plants exhibit Fibonacci numbers in their leaf patterns.
Some common trees with their Fibonacci leaf arrangement numbers are:
1/2 elm, linden, lime, grasses
1/3 beech, hazel, grasses, blackberry
2/5 oak, cherry, apple, holly, plum, common groundsel
3/8 poplar, rose, pear, willow
5/13 pussy willow, almond
The numerator is # of turns and denominator is # of leaves til we arrive a a leaf directly under the starting leaf. Each leaf is this fraction of a turn after the last leaf. Or, there are so many turns (numerator) for every so many leaves (denominator).
Cactus's spines often show the same spirals as we see on pine cones, petals and leaf arrangements, but they are much more clearly visible.
Plants illustrate the Fibonacci series in the numbers and arrangements of petals, leaves, sections and seeds. Plants that are formed in spirals, such as artichokes, pinecones, pineapples, daisies and sunflowers, illustrate Fibonacci numbers. Many plants produce new branches in quantities that are based on Fibonacci numbers.
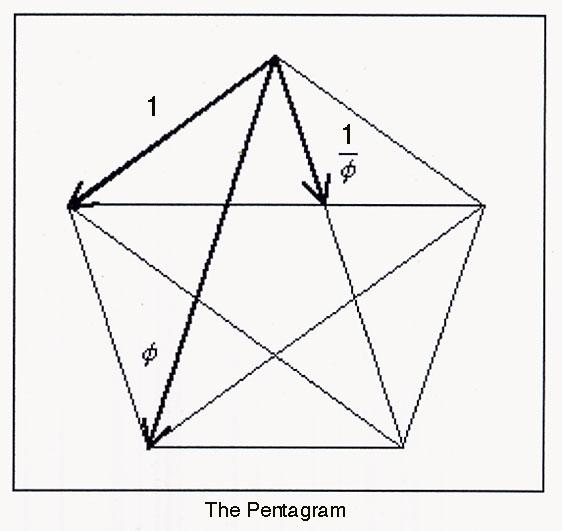
Calla Lilies have one petal. Euphorbia have two petals. Lilies have three. Buttercups, Roses, Pinks and Geraniums have five, Delphiniums and Bloodroot have 8 petals, Marigolds and Ragworts have 13, Asters and Black-eyed Susans 21, Pyrethrum have 34, and most Daisies have 34, 55, or 89. You don't find any other numbers very often. (The main exceptions are when those same numbers appear doubled, or when the so-called anomalous series appears: 3, 4, 7, 11, 18 and so on.). The five-pointed star is unusually common in nature. Not only in flowers, but also in the starfish, sand dollar, apple, etcetera. Why? Because Phi is the ratio of the side of a pentagon to its diagonal (see picture).
The Sunflower, Pinecone, Daisy and Nautilus Shell
Furthermore, the SUNFLOWER has 21 spirals on its head in one direction and 34 going in the other -- consecutive Fibonacci numbers. Or 34 and 55, or 55 and 89, or 89 and 144. The precise numbers depend on the species of SUNFLOWER.The same is true with DAISIES. The outside of a PINECONE has spirals that run clockwise and counterclockwise, and the ratio of the number of spirals is sequential Fibonacci values. In the elegant curves of a NAUTILUS shell, each complete new revolution will be a ratio of 1:1.618, when compared to the distance from the center of the previous spiral. If the divergence angle of a SUNFLOWER varies from 137.5 degrees (seen in the pattern below) to either 137.4 or 137.6 degrees, much of the beauty of the spiral is lost as well as its most compact arrangement. The SUNFLOWER therefore is in a precise state of perfection. ROMANESQUE BROCCOLI/CAULIFLOWER is Fibonacci spiral upon spiral. The same can be said of any other plant or animal containing this Fibonacci sequence. Everything in nature is already in a state of perfection -- contrary to the theory of Evolution..Where are the transitional stages? Where are the vestigial organs? Where are the imperfect spirals? Who told a DAISY to have the same Fibonacci sequence as a NAUTILUS, or a PINECONE, or a SUNFLOWER, or a RAM'S HORN or even the appearance of a BREAKING WAVE? None of them have a brain. None of them are in any way related to the other. Design demands a Designer. Beauty demands a Master Artist.
The Human Head
Human beauty is based on the Divine Proportion. Many experiments have been carried out to prove that the proportions of the top models' faces conform more closely to the Golden Ratio than the rest of the population. The human face is based entirely on Phi .The head forms a golden rectangle with the eyes at its midpoint. The mouth and nose are each placed at golden sections of the distance between the eyes and the bottom of the chin. The ear reflects the shape of a Fibonacci spiral. Even the dimensions of our teeth are based on phi. The front two incisor teeth form a golden rectangle, with a phi ratio in the heighth to the width. The ratio of the width of the first tooth to the second tooth from the center is also phi. The ratio of the width of the smile to the third tooth from the center is phi as well.
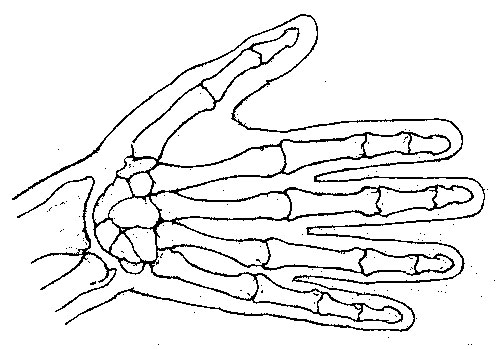
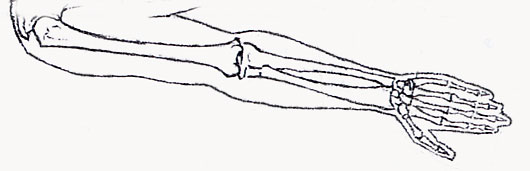
The Human Finger Joints and Hand
Each segment of each finger, from the tip to the base of the wrist, is larger than the preceding one by about the Fibonacci ratio of 1.618. In other words, the smallest segment is 2, the next 3, the next 5, and the back of your hand is 8. By this scale, your fingernail is 1 unit in length. You also have 2 hands, each with 5 digits, and your 8 fingers are each comprised of 3 sections. All Fibonacci numbers! Your hand creates a golden section in relation to your forearm, as the ratio of your forearm to your hand is also 1.618, the Divine Proportion. Furthermore, your hand and forearm together are a golden section in relation to the entire length of your arm.
The Human Body
The ventricles of the human heart reset themselves at the Golden Ratio point of the heart's rhythmic cycle.
Leonardo Da Vinci found that the total height of the human body, from toes to top of head, and the height from the toes to the navel depression are in Golden Ratio. Also the height from toes to navel and from the navel to the top of the head are in Golden Ratio. This has been confirmed by measuring 207 students at the Pascal Gymnasium in Munster Germany, where the perfect value of 1.618 was obtained for the first ratio average and 1.619 was obtained for the second. This value held for both girls and boys of similar ages ("Golden Mean of the Human Body" by T. Antony Davis and Rudolf Altevogt).
But there is more. From the top of the head to the bottom of the feet is the body's total height A. From the top of the head to the bottom of the fingertips is a golden section B of the total height A. From the top of the head to the navel and elbows is a golden section C of the golden section B. From the top of the head to the pectorals and armpits, the width of the shoulders, the length of the forearm and shinbone is a golden section D of the golden section C. From the top of the head to the base of the skull and the width of the abdomen is a golden section E of the golden section D. The width of the head and half the width of the chest and the hips is the golden section F of the golden section E.
The human body is also based on the number 5: The torso has 5 appendages, in the arms, legs and head., In turn, each of these has five appendages, in the fingers and toes and 5 openings on the face. and 5 senses in sight, sound, touch, taste and smell. The golden section is also based on 5, as the number phi, or 1.6180339 is computed from the square root of 5 multiplied by .5 plus .5 = Phi.
Relative Planetary Distances
The relative planetary distances average to Phi. The average of the mean orbital distances of each successive planet in relation to the one before it approximates phi: The mean distance is in million kilometers per NASA. If Mercury is 1, these are the relative mean distances Mercury 57.91 (1.00000) Venus 108.21 (1.86859) Earth 149.60 (1.38250) Mars 227.92 (1.52353) Ceres (the largest asteroid) 413.79 (1.81552) Jupiter 778.57 (1.88154) Saturn 1,433.53 (1.84123) Uranus 2,872.46 (2.00377) Neptune 4,495.06 (1.56488) and Pluto 5,869.66 (1.30580) The total is 16.18736 and so if we divide by 10, we get the average which is1.61874. Phi is1.61803.
The Fibonacci Series also predicts accurately the distances of the moons of Jupiter, Saturn and Uranus from each one's respective parent planet. Individual offsets can be attributed to planetary densities. Jupiter has 12 moons. Saturn has 9 moons and Uranus has 5 moons. For actual plotted graphs of these amazing results refer to the Fibonacci Quarterly October 1970, vol. 8, Number 4.
The Greatest Human Achievements Include the Golden Ratio
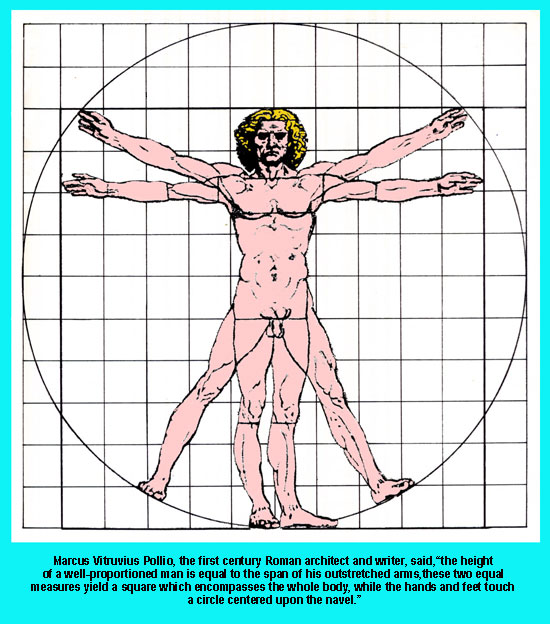
The dimensions of the King's Chamber of the Great Pyramid in Giza, Egypt are based upon the Golden Ratio. The Great Pyramid's vertical height and the width of any of its sides are in Golden Sections. The architect, Le Corbusier designed his Modulor system around the use of the ratio; the painter Mondrian based most of his work on the Golden Ratio; Leonardo Da Vinci included it in many of his paintings and Claude Debussy used its properties in his music. So did Bela Bartok. The Golden Ratio also is found in widescreen televisions, postcards, credit cards, and photographs which all commonly conform to its proportions. Like the brilliant Pythagoras before him, Leonardo had made an in-depth study of the human figure, showing how all of its major parts were related to the Golden Ratio. The Mona Lisa's face fits inside a perfect Golden Rectangle. The Golden Rectangle is found in his painting called The Last Supper also. Many other Renaissance artists did the same. After Leonardo, artists such as Raphael and Michelangelo made great use of the Golden Ratio to construct their works. Michelangelo's beautiful sculpture of David conforms to the Golden Ratio, from the location of the navel with respect to the height and placement of the joints in the fingers. The builders of the medieval and Gothic churches and cathedrals of Europe also made these structures conform to the Golden Ratio. The Golden Rectangle is one whose sides are in the proportion of the Golden Ratio. This means the longer side is 1.618 times longer than the shorter side. The front of the Parthenon in Athens, Greece can be framed within a Golden Rectangle. Da Vinci's drawing of the Vitruvian Man has the outlines of a rectangle based on the head, one on the torso, and another over the legs.
The Golden Ratio In The Bible
The Ark of the Covenant is a Golden Rectangle. In Exodus 25:10, God commands Moses to build the Ark of the Covenant, in which to hold His Covenant with the Israelites, the Ten Commandments, saying,"Have them make a chest of acacia wood -- two and a half cubits long, a cubit and a half wide, and a cubit and a half high." The ratio of 2.5 to 1.5 is 1.666..., which is as close to phi (1.618 ...) as you can come with such simple numbers and is certainly not visibly different to the eye. The Ark of the Covenant is thus constructed using the Golden Section, or Divine Proportion. This ratio is also the same as 5 to 3, numbers from the Fibonacci series.
Noah's Ark uses a Golden Rectangle. In Genesis 6:15, God commands Noah to build an ark saying,"And this is the fashion which thou shalt make it of: The length of the ark shall be three hundred cubits, the breadth of it fifty cubits, and the height of it thirty cubits."Thus the end of the ark, at 50 by 30 cubits, is also in the ratio of 5 to 3, or 1.666..., again a close approximation of phi not visibly different to the naked eye. Noah's ark was built in the same proportion as ten arks of the covenant placed side by side.
Exodus 27:1-2 mentions the dimensions of the altar -- constructed according to phi: "Build an altar of acacia wood, three cubits high; it is to be square, five cubits long and five cubits wide."
Furthermore, the location of Jerusalem is 31 degrees 45 minutes north of the equator. God said Jerusalem is "the city which I have chosen to put my name there" (1 Kings 11:36). Why did God select this location? First build a rectangular building in Jerusalem with sides that exhibit the golden rectangle ratio. The longer two sides (1.618) must run from east to west. The shorter two sides (1) must run from north to south. Then at each of the four corners place a flag pole. Make the roof flat. Remember that the sun rises in the east and sets in the west. Now if you draw one diagonal line from the northwest to the southeast corner, and another diagonal line from the northeast to southwest corner, you will create angles of 31 degrees 45 minutes with respect to the straight line joining the two southern corners of the building. At the summer solstice, the sun is over the Tropic of Cancer, 23.5 degrees north of the equator. At this point the shadows will point most easterly and westerly. At the winter solstice, the sun is over the Tropic of Capricorn, 23.5 degrees south of the equator. At this point the shadows will point most northerly. On the spring (March 21) and fall (Sept. 23) equinoxes, when the sun is directly over the equator, the shadows created by sunrise and sunset will fall exactly on these diagonal lines. This method can be used at any latitude with rectangles of different proportions. But a rectangle with the proportions of "the golden section " will only give this result at the exact latitude of Jerusalem. It is important to know when the equinoxes occur each year in order to celebrate God's festivals. We are to take the first new moon on or after the spring equinox as New Year's Day. No complex postponement rules or calculated calendar is necessary. Babylon is about one degree further north than Jerusalem. The pyramids of Gizeh are further south and Mecca still further south.

Fibonacci Numbers In The Musical Scale
The musical scales are based on Fibonacci numbers. The piano keyboard scale of C to C has 8 white keys in an "octave" and 5 black keys, making 13 keys total. The 5 black keys are divided into groups: of 2 keys and 3 keys. A scale is comprised of 8 notes, of which the 5th and 3rd notes create the basic foundation of all chords, and are based on whole tone which is 2 steps from the root tone, that is the1st note of the scale. Although there are only 12 notes in the scale, if you don't have a root and octave, a start and an end, you have no means of calculating the gradations in between, so this 13th note as the octave is essential to computing the frequencies of the other notes. The word "octave" comes from the Latin word for 8, referring to the eight whole tones of the complete musical scale, which in the key of C are C-D-E-F-G-A-B-C.
In a scale, the dominant note is the 5th note of the major scale, which is also the 8th note of all 13 notes that comprise the octave. This provides another instance of Fibonacci numbers in key musical relationships. Interestingly, 8/13 is .61538, which approximates phi. What's more, the typical three chord song in the key of A is made up of A, its Fibonacci & phi partner E, and D, to which A bears the same relationship as E does to A. This is analogous to the "A is to B as B is to C" basis for the golden section, or in this case "D is to A as A is to E."
Musical frequencies are based on Fibonacci ratios Notes in the scale of western music have a foundation in the Fibonacci series, as the frequencies of musical notes have relationships based on Fibonacci numbers: A440 Hertz is an arbitrary standard. The American Federation of Musicians accepted the A440 as standard pitch in 1917. It was then accepted by the U.S. government its standard in 1920 and it was not until 1939 that this pitch was accepted internationally. Before recent times a variety of tunings were used. It has been suggested by James Furia and others that A432 be the standard. A432 was often used by classical composers and results in a tuning of the whole number frequencies that are connected to numbers used in the construction of a variety of ancient works and sacred sites, such as the Great Pyramid of Egypt. The controversy over tuning still rages, with proponents of A432 or C256 as being more natural tunings than the current standard. |
|
|
|
جواب |
رسائل 33 من 166 في الفقرة |
|
|
Posted on viernes, 15 de enero de 2010 20:55:47 by truthfinder9
Interesting. From this book (sorry, not all of the formulas converted right):
Mathematical Signatures in Nature: A Sign of Design?
“[The Universe] is written in the language of mathematics, and its characters are triangles, circles, and other geometrical figures, without which it is humanly impossible to understand a single word of it...” – Galileo Galilei1
Math is the universal language, but it is not a human construct. Sure, we create symbols for numbers and mathematical computations, but math itself is more fundamental. 2 + 2 = 4 is universally true, universal in the sense that everywhere in the universe it is true. It does not matter what symbols we create to communicate “2 + 2 = 4” — the equation always remains true. In other words, what we call math is simply a way to describe or visualize the order that is the foundation of the universe’s structure and mechanics.
As we have discussed at length, such order cannot be produced by chance. The level of order is too sophisticated for a random cause. The patterns that are often revealed are too precise. Only intelligence produces such things. Let us look at some of the mathematical constructs that we have uncovered in nature.
Phi [M]
Phi (M = 1.6180339887...) is an irrational number like pi (p = 3.141592653...). Phi and pi are both ratios defined by particular Euclidean geometries, with phi being the division of a line “so that the ratio of the lesser part to the greater part is the same as the ratio of the greater part to the whole.2”
Phi’s abundance in the universe as earned it names such as the Golden Section, the Divine Proportion, the Golden Ratio and the Golden Mean. These names stem from the fact that phi can be found in many natural constructs such as in human and animal proportions (i.e. the arrangement of physical features). Phi relationships can be found in DNA, among the planets of the Solar System (as in Kepler’s Laws), and so on. Even in fractal geometry (used for the irregular geometries found in nature) we find phi in everything from coastlines to crystal formation.
Many argue that phi is also used by humans in such things as art, architecture and music for the balance it produces in designs. However, there are some caveats to this. For example, some claim one can find phi — not surprisingly — in the structure or design of the pyramids. Is phi intentional in the pyramids or merely the result of what is good geometrical design? It is probably the latter. Architecture is often designed with balance and stability which necessitates geometries that contain phi, though these geometries are not always necessary. Phi may be intentional in some structures, but it is accidental or inadvertent in others.
While there is always an amount of subjectivity in how one designs a structure, there is much more of this in artwork. Phi can be seen in certain art pieces, but many more do not exhibit phi. Art that uses geometry as a basis will naturally converge on phi (with phi not necessarily “encoded” in the painting by the artist). We can purposely incorporate phi or inadvertently do so through our use of the geometries we have discovered.
Either way we (intelligent designers) are using precise constructs. How do such precise relations appear repeatedly in nature?
Fibonacci Series
This is the Fibonacci series: 0, 1, 1, 2, 3, 5, 8, 13, 21, 34. . .etc. It is very simply explained as each number in the series being the sum of the previous two numbers. The ratio of each successive pair of numbers (starting with 3/2) in the series approximates phi (i.e. 8 divided by 5 is 1.6). The accuracy of these ratios’ approximations to phi increases considerably as you go through the series. Phi can also be used to estimate any number in the Fibonacci series: fn = Mn / 5½.
Why is this important? Because the Fibonacci series precisely describes the spiral patterns common in nature: In shells, hurricanes, whirlpools, spiral galaxies, DNA and plant life. Phi is all around us. For example: The ratio of scales in the opposing spirals around a pine cone is 5:8; bumps on a pineapple are 8:13; seeds in a sunflower are 21:34. All of these ratios are adjacent pairs in the Fibonacci series.
Biblical Indications of Phi?
Exodus 25:10 writes: “Have them make a chest of acacia wood — two and a half cubits long, a cubit and a half wide, and a cubit and a half high.” Here we find that “The ratio of 2.5 to 1.5 is 1.666..., which is as close to phi (1.618...) as you can come with such simple numbers and is certainly not visibly different to the eye. The Ark of the Covenant is thus constructed using the Golden Section, or Divine Proportion. This ratio is also the same as 5 to 3, numbers from the Fibonacci series.3”
God instructed Noah in Genesis 6:15 to build an ark this way: “This is how you are to build it: The ark is to be 450 feet long, 75 feet wide and 45 feet high.” Hence, 75 by 45 feet is also in the ratio of 5 to 3, or 1.666..., another “close approximation of phi not visibly different to the naked eye. Noah’s ark was built in the same proportion as ten arks of the covenant placed side by side.4”
These indications of phi may or may not have been intentional. However, they are more evidence of a trend for man to use the same logic embedded in the universe. As Gary Meisner writes, “The pervasive appearance of phi throughout life and the universe is believed by some to be the signature of God, a universal constant of design used to assure the beauty and unity of His creation.5”
Pi [p]
Pi is the ratio of the circumference of a circle to its diameter (p = 3.141592653...). Pi is just as fundamental as phi in the universe, but because it is more familiar, its presence does not seem as startling. Yet mathematicians have spent millennia computing its numbers and looking for patterns in the pi sequence. Perhaps not surprisingly, pi can be related to phi. One way is through trigonometric relations: 2 H cos (p / 5) = M and 2 H sin (p / 5) = Ö(3 - M). There are other relations as well.6
Pi in the Bible?
1 Kings 7:23 writes: “He made the Sea of cast metal, circular in shape, measuring ten cubits from rim to rim and five cubits high. It took a line of thirty cubits to measure around it.” We know that circumference equals pi times diameter (c = pd). So from 1 Kings 7:23 we produce 30 = p10. Solving for p we get 3. Since p actually equals 3.141592653..., some people reason that the Bible is wrong. Is it?
First we must recognize that the Bible commonly uses rounded figures. These are descriptions, not architectural blueprints. Secondly, it has been shown by some that since the Hebrew does not have digits — all letters are also numbers — the relevant Hebrew in this passage can be calculated to find pi.7 The calculation comes out to 3.14150943... This is only a difference of 0.0000832 with actual pi, making the Bible’s description of pi the most accurate in antiquity.
Perhaps the Hebrews did not specifically calculate pi, but they managed to come very close “accidentally.” Another caveat is that many have tried to abuse the Hebrew and find endless “codes” hidden in the Bible. It can be shown how and why these codes are nonexistent8 or at least cannot be attributed the variety of meanings that some people claim the codes produce. While certain numbers, sequences, etc., in the Bible and elsewhere have been attributed particular meanings and usages, there is a big difference between what can be defined as pseudoscientific numerologies and scientific patterns. The latter are constant and repeatable (pi and phi) the former are inconsistent and variable (“Bible codes”).
Universal Language
So it seems mathematical patterns are inherent to the very structure of the universe. While we use our language of mathematics to describe these patterns and all of the precise fine-tuning found in the universe, the mathematical ratios themselves seem to be design evidences: A universal language, unchanging throughout time or place; too precise and nonnrandom to be products of chance.
We have also seen how biblical writings show examples of these mathematical constructs, both in usage and understanding. Once again the Bible shows a level of knowledge and accuracy that matches and surpasses other cultures contemporary to the Hebrews.
1. Mario Livio, The Golden Ratio (New York, NY: Broadway, 2003), pp. 241-242.
2. Miranda Lundy, Sacred Geometry (New York. NY: Walker, 1998), pp. 24-25.
For more on how phi is defined, see the websites “Phi: The Golden Number” at www.goldennumber.net by Gary Meisner, “The Golden section ratio: Phi” at www.mcs.surrey.ac.uk/Personal/R.Knott/Fibonacci/phi.html by Ron Knott and “Phi: That Golden Number” at jwilson.coe.uga.edu/emt669/Student.Folders/Frietag.Mark/Homepage/Goldenratio/goldenratio.html by Mark Freitag.
3. - 5. Gary Meisner’s “Phi in the Bible” at goldennumber.net/bible.htm.
6. Gary Meisner, “Pi, Phi and Fibonacci Numbers” at goldennumber.net/pi-phi-fibonacci.htm. More on the Fibonacci series in Ron Knott’s “Fibonacci Numbers and the Golden Section” at www.mcs.surrey.ac.uk/Personal/R.Knott/Fibonacci/fib.html.
7. Jochen Katz, “Pi in the Bible” at answering-islam.org.uk/Religions/Numerics/pi.html.
8. Randall Ingermanson, Who Wrote the Bible Code? A Physicist Probes the Current Controversy. Colorado Springs, Colorado: Waterbook, 1999. The author maintains additional information and appendices to his book on-line at www.rsingermanson.com. More on the Bible codes can be found at www.reasons.org/resources/apologetics/biblecode.shtml?main and at www.answering-islam.org.uk/Religions/Numerics/.
http://www.freerepublic.com/focus/f-religion/2429476/posts |
|
|
|
جواب |
رسائل 34 من 166 في الفقرة |
|
The Fibonacci Sequence
Studied along time ago, God's numbers.People love to leave God out of these numbers,study the human body-ALL PROPORTIONS ARE GODS NUMBERS.Fingers, to toes, to nose.A starter;
Another notable example is human body.In human body, the ratio of the length of forearm to the length of the hand is equal to 1.618, that is, Golden Ratio. Another well-known examples on human body are:
The ratio between the length and width of face
Ratio of the distance between the lips and where the eyebrows meet to the length of nose
Ratio of the length of mouth to the width of nose
Ratio of the distance between the shoulder line and the top of the head to the head length
Ratio of the distance between the navel and knee to the distance between the knee and the end of the foot
Ratio of the distance between the finger tip and the elbow to the distance between the wrist and the elbow
Read more: http://scienceray.com/mathematics/th...#ixzz0vyU32DdS
---------------------
Fibonacci Numbers and Nature
This page has been split into TWO PARTS.
This, the first, looks at the Fibonacci numbers and why they appear in various "family trees" and patterns of spirals of leaves and seeds.
The second page then examines why the golden section is used by nature in some detail, including animations of growing plants.
http://www.maths.surrey.ac.uk/hosted...ci/fibnat.html
---------------------------------------------------------
The Human Head
Human beauty is based on the Divine Proportion. Many experiments have been carried out to prove that the proportions of the top models' faces conform more closely to the Golden Ratio than the rest of the population. The human face is based entirely on Phi .The head forms a golden rectangle with the eyes at its midpoint. The mouth and nose are each placed at golden sections of the distance between the eyes and the bottom of the chin. The ear reflects the shape of a Fibonacci spiral. Even the dimensions of our teeth are based on phi. The front two incisor teeth form a golden rectangle, with a phi ratio in the heighth to the width. The ratio of the width of the first tooth to the second tooth from the center is also phi. The ratio of the width of the smile to the third tooth from the center is phi as well.
The Human Finger Joints and Hand
Each segment of each finger, from the tip to the base of the wrist, is larger than the preceding one by about the Fibonacci ratio of 1.618. In other words, the smallest segment is 2, the next 3, the next 5, and the back of your hand is 8. By this scale, your fingernail is 1 unit in length. You also have 2 hands, each with 5 digits, and your 8 fingers are each comprised of 3 sections. All Fibonacci numbers! Your hand creates a golden section in relation to your forearm, as the ratio of your forearm to your hand is also 1.618, the Divine Proportion. Furthermore, your hand and forearm together are a golden section in relation to the entire length of your arm.
The Human Body
The ventricles of the human heart reset themselves at the Golden Ratio point of the heart's rhythmic cycle.
Leonardo Da Vinci found that the total height of the human body, from toes to top of head, and the height from the toes to the navel depression are in Golden Ratio. Also the height from toes to navel and from the navel to the top of the head are in Golden Ratio. This has been confirmed by measuring 207 students at the Pascal Gymnasium in Munster Germany, where the perfect value of 1.618 was obtained for the first ratio average and 1.619 was obtained for the second. This value held for both girls and boys of similar ages ("Golden Mean of the Human Body" by T. Antony Davis and Rudolf Altevogt).
But there is more. From the top of the head to the bottom of the feet is the body's total height A. From the top of the head to the bottom of the fingertips is a golden section B of the total height A. From the top of the head to the navel and elbows is a golden section C of the golden section B. From the top of the head to the pectorals and armpits, the width of the shoulders, the length of the forearm and shinbone is a golden section D of the golden section C. From the top of the head to the base of the skull and the width of the abdomen is a golden section E of the golden section D. The width of the head and half the width of the chest and the hips is the golden section F of the golden section E.
The human body is also based on the number 5: The torso has 5 appendages, in the arms, legs and head., In turn, each of these has five appendages, in the fingers and toes and 5 openings on the face. and 5 senses in sight, sound, touch, taste and smell. The golden section is also based on 5, as the number phi, or 1.6180339 is computed from the square root of 5 multiplied by .5 plus .5 = Phi.
Relative Planetary Distances
The relative planetary distances average to Phi. The average of the mean orbital distances of each successive planet in relation to the one before it approximates phi: The mean distance is in million kilometers per NASA. If Mercury is 1, these are the relative mean distances Mercury 57.91 (1.00000) Venus 108.21 (1.86859) Earth 149.60 (1.38250) Mars 227.92 (1.52353) Ceres (the largest asteroid) 413.79 (1.81552) Jupiter 778.57 (1.88154) Saturn 1,433.53 (1.84123) Uranus 2,872.46 (2.00377) Neptune 4,495.06 (1.56488) and Pluto 5,869.66 (1.30580) The total is 16.18736 and so if we divide by 10, we get the average which is1.61874. Phi is1.61803.
The Fibonacci Series also predicts accurately the distances of the moons of Jupiter, Saturn and Uranus from each one's respective parent planet. Individual offsets can be attributed to planetary densities. Jupiter has 12 moons. Saturn has 9 moons and Uranus has 5 moons. For actual plotted graphs of these amazing results refer to the Fibonacci Quarterly October 1970, vol. 8, Number 4.
The Greatest Human Achievements Include the Golden Ratio
The dimensions of the King's Chamber of the Great Pyramid in Giza, Egypt are based upon the Golden Ratio. The Great Pyramid's vertical height and the width of any of its sides are in Golden Sections. The architect, Le Corbusier designed his Modulor system around the use of the ratio; the painter Mondrian based most of his work on the Golden Ratio; Leonardo Da Vinci included it in many of his paintings and Claude Debussy used its properties in his music. So did Bela Bartok. The Golden Ratio also is found in widescreen televisions, postcards, credit cards, and photographs which all commonly conform to its proportions. Like the brilliant Pythagoras before him, Leonardo had made an in-depth study of the human figure, showing how all of its major parts were related to the Golden Ratio. The Mona Lisa's face fits inside a perfect Golden Rectangle. The Golden Rectangle is found in his painting called The Last Supper also. Many other Renaissance artists did the same. After Leonardo, artists such as Raphael and Michelangelo made great use of the Golden Ratio to construct their works. Michelangelo's beautiful sculpture of David conforms to the Golden Ratio, from the location of the navel with respect to the height and placement of the joints in the fingers. The builders of the medieval and Gothic churches and cathedrals of Europe also made these structures conform to the Golden Ratio. The Golden Rectangle is one whose sides are in the proportion of the Golden Ratio. This means the longer side is 1.618 times longer than the shorter side. The front of the Parthenon in Athens, Greece can be framed within a Golden Rectangle. Da Vinci's drawing of the Vitruvian Man has the outlines of a rectangle based on the head, one on the torso, and another over the legs.
The Golden Ratio In The Bible
The Ark of the Covenant is a Golden Rectangle. In Exodus 25:10, God commands Moses to build the Ark of the Covenant, in which to hold His Covenant with the Israelites, the Ten Commandments, saying,"Have them make a chest of acacia wood -- two and a half cubits long, a cubit and a half wide, and a cubit and a half high." The ratio of 2.5 to 1.5 is 1.666..., which is as close to phi (1.618 ...) as you can come with such simple numbers and is certainly not visibly different to the eye. The Ark of the Covenant is thus constructed using the Golden Section, or Divine Proportion. This ratio is also the same as 5 to 3, numbers from the Fibonacci series.
Noah's Ark uses a Golden Rectangle. In Genesis 6:15, God commands Noah to build an ark saying,"And this is the fashion which thou shalt make it of: The length of the ark shall be three hundred cubits, the breadth of it fifty cubits, and the height of it thirty cubits."Thus the end of the ark, at 50 by 30 cubits, is also in the ratio of 5 to 3, or 1.666..., again a close approximation of phi not visibly different to the naked eye. Noah's ark was built in the same proportion as ten arks of the covenant placed side by side.
Exodus 27:1-2 mentions the dimensions of the altar -- constructed according to phi: "Build an altar of acacia wood, three cubits high; it is to be square, five cubits long and five cubits wide."
Furthermore, the location of Jerusalem is 31 degrees 45 minutes north of the equator. God said Jerusalem is "the city which I have chosen to put my name there" (1 Kings 11:36). Why did God select this location? First build a rectangular building in Jerusalem with sides that exhibit the golden rectangle ratio. The longer two sides (1.618) must run from east to west. The shorter two sides (1) must run from north to south. Then at each of the four corners place a flag pole. Make the roof flat. Remember that the sun rises in the east and sets in the west. Now if you draw one diagonal line from the northwest to the southeast corner, and another diagonal line from the northeast to southwest corner, you will create angles of 31 degrees 45 minutes with respect to the straight line joining the two southern corners of the building. At the summer solstice, the sun is over the Tropic of Cancer, 23.5 degrees north of the equator. At this point the shadows will point most easterly and westerly. At the winter solstice, the sun is over the Tropic of Capricorn, 23.5 degrees south of the equator. At this point the shadows will point most northerly. On the spring (March 21) and fall (Sept. 23) equinoxes, when the sun is directly over the equator, the shadows created by sunrise and sunset will fall exactly on these diagonal lines. This method can be used at any latitude with rectangles of different proportions. But a rectangle with the proportions of "the golden section " will only give this result at the exact latitude of Jerusalem. It is important to know when the equinoxes occur each year in order to celebrate God's festivals. We are to take the first new moon on or after the spring equinox as New Year's Day. No complex postponement rules or calculated calendar is necessary. Babylon is about one degree further north than Jerusalem. The pyramids of Gizeh are further south and Mecca still further south.
Fibonacci Numbers In The Musical Scale
The musical scales are based on Fibonacci numbers. The piano keyboard scale of C to C has 8 white keys in an "octave" and 5 black keys, making 13 keys total. The 5 black keys are divided into groups: of 2 keys and 3 keys. A scale is comprised of 8 notes, of which the 5th and 3rd notes create the basic foundation of all chords, and are based on whole tone which is 2 steps from the root tone, that is the1st note of the scale. Although there are only 12 notes in the scale, if you don't have a root and octave, a start and an end, you have no means of calculating the gradations in between, so this 13th note as the octave is essential to computing the frequencies of the other notes. The word "octave" comes from the Latin word for 8, referring to the eight whole tones of the complete musical scale, which in the key of C are C-D-E-F-G-A-B-C.
In a scale, the dominant note is the 5th note of the major scale, which is also the 8th note of all 13 notes that comprise the octave. This provides another instance of Fibonacci numbers in key musical relationships. Interestingly, 8/13 is .61538, which approximates phi. What's more, the typical three chord song in the key of A is made up of A, its Fibonacci & phi partner E, and D, to which A bears the same relationship as E does to A. This is analogous to the "A is to B as B is to C" basis for the golden section, or in this case "D is to A as A is to E."
Musical frequencies are based on Fibonacci ratios Notes in the scale of western music have a foundation in the Fibonacci series, as the frequencies of musical notes have relationships based on Fibonacci numbers: A440 Hertz is an arbitrary standard. The American Federation of Musicians accepted the A440 as standard pitch in 1917. It was then accepted by the U.S. government its standard in 1920 and it was not until 1939 that this pitch was accepted internationally. Before recent times a variety of tunings were used. It has been suggested by James Furia and others that A432 be the standard. A432 was often used by classical composers and results in a tuning of the whole number frequencies that are connected to numbers used in the construction of a variety of ancient works and sacred sites, such as the Great Pyramid of Egypt. The controversy over tuning still rages, with proponents of A432 or C256 as being more natural tunings than the current standard.
Musical compositions often reflect Fibonacci numbers and phi Fibonacci and phi relationships are often found in the timing of musical compositions. As an example, the climax of songs is often found at roughly the phi point (61.8%) of the song, as opposed to the middle or end of the song. In a 32 bar song, this would occur in the 20th bar.
If you'd like to know more, we publish a 32-page booklet called Who Is The Scarlet Harlot of Babylon? ($3.00). Use this link to pay with a credit card:
- US orders ($0.50 shipping)
- Canada/Mexico orders ($0.60 shipping)
http://www.goldismoney2.com/showthread.php?7277-The-Fibonacci-Sequence
|
|
|
|
جواب |
رسائل 35 من 166 في الفقرة |
|

from Mid-Atlantic Geomancy
If we want to talk with God/dess, experience has shown that it helps to be in the right environment. Spiritual seekers from Mayans through Christians, Native Americans, Egyptians and Hindus to the Neolithic builders of the stone rings in Britain and Ireland (and many more) found that by constructing their sacred places using certain geometrical ratios - just a small handful of them - they could more easily connect with their Maker.
Yes, it is possible to speak with our Creator anytime. However, sacred geometry makes this easier, and different ratios make different connections easier. The ratios have to do with different spiritual activities like healing, foretelling the future, long-distance communication, levitation and, most important, heightened ability to communicate with our Maker. These ratios help us to vibrate at the appropriate frequency to aid us in accomplishing the particular spiritual activity we have in mind.
Nearly every ancient archaeological site predating recorded history, from the Pyramids of Egypt and Mexico, to Stonehenge and beyond, employs mysterious mathematical alignments throughout their design.
These architectural formulas, rarely used today, are considered sacred and have also been found in the way they're arranged relation to each other and, most inexplicably, in the Monuments of Cydonia, and the Face on Mars.
When one looks at sacred enclosures globally, there is a group of five mathematical ratios that are found all over the world from Japan's pagodas to Mayan temples in the Yucatan, and from Stonehenge to the Great Pyramid. These ratios are:
-
Square Root of Two = 1.414...
-
Square Root of Three = 1.732...
-
Square Root of Five = 2.236...
-
Phi = 1.618...
Phi is the Golden Section of the Greeks. It was said to be the first section in which the One became many.
-
Pi = 3.1416...
Pi is found in any circle. If the diameter is 1, the circumference is 3.1416 (C = D).
These are all irrational numbers. Pi can be taken to 1500 decimal places with no discernable pattern to it (is that Chaos?). Let's take a closer look at each of these special numbers, and see how we can find them in the sacred geometry used by geomancers around the world. All five of these numbers gain their meaning only when beaten against the One. They are all ratios of x:1. The One is where it begins.
Pi - 3.1416 : 1 - the Circle
|
Pi (3.1416 : 1) is found in any circle. In sacred geometry, the circle represents the spiritual realms.
A circle, because of that transcendental number pi, cannot be described with the same degree of accuracy as the physical square.
The circle is yin.
It is a good shape to do all kinds of spiritual activities in. It is good for groups to work in circles. There are many examples of sacred spaces that are circular.
|
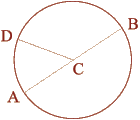
The Circle:
Radius (CD) = 1
Diameter (AB) = 2
Circumference = pi (3.1416) x Diameter
|
|

Ring of Brodgar, Mainland Orkney.
|
Most stone rings in the British isles are not actually circular.
Dr Alexander Thom proved this with his pioneering work in the sixties.
Some of the true circles are Merry Maidens in Cornwall, Stonehenge and the Ring of Brodgar.
|
Square Root of Two - 1.414 : 1 - the Square
|
In sacred geometry, the square represents the physical world.
It can be defined totally.
If its side is one, its perimeter is exactly four, and its area is one square - exactly.
The Square is yang.
|
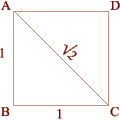
The Square Side (AB) = 1
Diagonal (AC) = Square Root of Two, 1.414
|
|
The Square Side (AB) = 1
Diagonal (AC) = Square Root of Two, 1.414
|
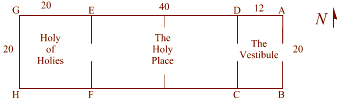
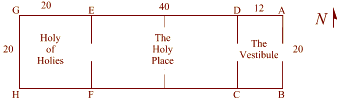
The square was found was in the Holy of Holies (the back room) of Solomon's Temple (G,H,F,E).
This was where the Hebrews kept the Ark of the Covenant and other most sacred treasures. (The dimensions here are taken from the first part of the Ezekiel Chapter 41.)
|
|
On top of if(screen) { topPos=0; leftPos=0; } // Open full page // // Open to size // var newWin1=null; function getPage(thePage,wt,ht) { leftPos= (screen.width-wt)/2; topPos = (screen.height-ht)/2; if ( newWin1 && !newWin1.closed ) { newWin1.close(); } newWin1 = window.open(thePage,'aWin','toolbars=no, resizeable=no,scrollbars=no,left='+leftPos+',top='+topPos+',width='+wt+',height='+ht); } // -->Glastonbury Tor sits an impressive stone tower.
The Tor and its tower dominate the if(screen) { topPos=0; leftPos=0; } // Open full page // // Open to size // var newWin1=null; function getPage(thePage,wt,ht) { leftPos= (screen.width-wt)/2; topPos = (screen.height-ht)/2; if ( newWin1 && !newWin1.closed ) { newWin1.close(); } newWin1 = window.open(thePage,'aWin','toolbars=no, resizeable=no,scrollbars=no,left='+leftPos+',top='+topPos+',width='+wt+',height='+ht); } // -->Somerset Levels .
This is a view taken from inside the tower looking upward.

|

|
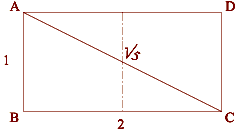
Square Root of Three - 1.732 : 1 - Vesica Pisces
The Vesica Pisces is created by two identical intersecting circles, the circumference of one intersecting the center of the other. The vulva-shaped space thus created is called the Vesica Pisces.
|

|
The Vesica Pisces:
Two Circles share a common radius (AB).
Radius AB = 1
The intersecting circles create a Vesica Pisces.
The minor axis of this Vesica Pisces (AB) = 1,
The major axis (CD) = the square root of three, 1.732
|
CB = AB
|
=
|
1 ..... Therefore:
|
|
a² + b²
|
=
|
c²
|
|
.5² + x²
|
=
|
1²
|
|
x²
|
=
|
.75
|
|
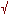 2 2
|
=
|
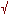 .75 .75
|
|
x
|
=
|
.8660 = CE
|
|
CE is 1/2 of the major axis CD
|
|
2 CE
|
=
|
CD
|
|
.8660 * 2
|
=
|
CD
|
|
CD
|
=
|
1.7320 =  3 3
|
|
|
This is the lid of the if(screen) { topPos=0; leftPos=0; } // Open full page // // Open to size // var newWin1=null; function getPage(thePage,wt,ht) { leftPos= (screen.width-wt)/2; topPos = (screen.height-ht)/2; if ( newWin1 && !newWin1.closed ) { newWin1.close(); } newWin1 = window.open(thePage,'aWin','toolbars=no, resizeable=no,scrollbars=no,left='+leftPos+',top='+topPos+',width='+wt+',height='+ht); } // -->Chalice Well designed by Bligh Bond in the early part of this century.
It covers one of the most powerful Holy Wells in Britain.
The Chalice Well has numerous examples of vesicas.
|

|
|

Gothic arch on the tower on the Glastonbury Tor. This site was a hermitage and retreat for early Christian monks
|

Gothic arch in Gallilee of Glastonbury Abbey. Note circular Romanesque arches behind in the Mary Chapel.
|
The top half of the Vesica Pisces is the Gothic Arch - see if(screen) { topPos=0; leftPos=0; } // Open full page // // Open to size // var newWin1=null; function getPage(thePage,wt,ht) { leftPos= (screen.width-wt)/2; topPos = (screen.height-ht)/2; if ( newWin1 && !newWin1.closed ) { newWin1.close(); } newWin1 = window.open(thePage,'aWin','toolbars=no, resizeable=no,scrollbars=no,left='+leftPos+',top='+topPos+',width='+wt+',height='+ht); } // -->Chartres Cathedral .
It is the sacred geometric shape of the Piscean Age.
|
Square Root of Five - 2.236 : 1 - the Double Square
|
The Double Square is found in some of the best known sacred spaces in the world, from the King's Chamber in the Great Pyramid and Solomon's Temple in the Bible to the interior of if(screen) { topPos=0; leftPos=0; } // Open full page // // Open to size // var newWin1=null; function getPage(thePage,wt,ht) { leftPos= (screen.width-wt)/2; topPos = (screen.height-ht)/2; if ( newWin1 && !newWin1.closed ) { newWin1.close(); } newWin1 = window.open(thePage,'aWin','toolbars=no, resizeable=no,scrollbars=no,left='+leftPos+',top='+topPos+',width='+wt+',height='+ht); } // -->Calendar II, an important underground stone chamber in Vermont, USA.
The diagonal of a double square is to the shorter side as the square root of five is to one.
The square root of five = .618 + 1 + .618.
|
The Double Square:
Short Side = 1
Longer Side = 2
Diagonal = Square Root of Five, 2.236
|
|
(ABCD) Double Square in Solomons Temple
|
if(screen) { topPos=0; leftPos=0; } // Open full page // // Open to size // var newWin1=null; function getPage(thePage,wt,ht) { leftPos= (screen.width-wt)/2; topPos = (screen.height-ht)/2; if ( newWin1 && !newWin1.closed ) { newWin1.close(); } newWin1 = window.open(thePage,'aWin','toolbars=no, resizeable=no,scrollbars=no,left='+leftPos+',top='+topPos+',width='+wt+',height='+ht); } // -->Solomons temple provides numerous examples of sacred geometry.
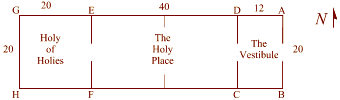
The holy place (EFCD) is the place where good Jews who had been properly cleansed could go.
This space measures twenty cubits by forty cubits.
|
Another place where a double square is found is the Calendar II underground chamber site in central Vermont in the USA. It measures ten feet by twenty feet.
|

.
Calendar II, a drystone walled underground stone chamber in central Vermont, USA.
|

The interior of the chamber is 20 feet long by 10 feet wide , or 2 to 1. The chamber is oriented towards the Winter Solstice Sunrise.
|
Phi - 1.618:1 - Ø - the Phi Rectangle
|
The Golden Section, Phi, 1.618:
The shorter section on the right = 3
The longer section = 5
The shorter is to the longer
as the longer is to the whole
3:5 : : 5:8
|
In the Beginning was the One. In order to observe itself, it cut part of itself away to make 'Other'. This Golden Section is in beautiful proportion. As the subdividing continued away from the One, they continued in this phi ratio.
This can be used to go back to the One as well. It is in this sense that three is farther away from the One than two is.
Have you ever noticed that it is easier mathematically to go away from One than to go towards it? In other words, it is easier to add and multiply than it is to subtract and divide.
|
3:5 : : 5:8. This ratio indicates that it is part of this series: 1 . 2 . 3 . 5 . 8 . 13 . 21 . 34 . 55 . 89, and so on. This is called the Fibonacci Series. Start anywhere in the series, add the number below, and you get the next number (for example, 21 + 13 = 34). As one ascends up the series, any number in the series, when divided into the next one up, gets closer and closer to (but never hits exactly) 1.618, phi, the Golden Section.
|
On a line create square
(ABCD) where AB = 1
|
|
|
Divide lines (AD) and (BC) in half
at (F) and (E). (BC) = 1, (EC) = .5
Double square (ECDF) is thus
created with a diameter of (ED).
|
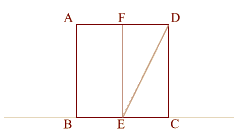
|
|
Using (ED) as a radius
swing arc from (D) downwards to 0
intersect the initial base line at (G).
Extend line (AFD), and create a
perpendicular to line (BECG) at (G)
so that it intersects line (AFD) at (H),
thus creating phi rectangle (ABGH).
|
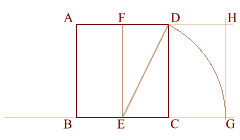
|
|
The formula that shows this is:
Phi = ( 1 + square root of 5 ) divided by 2
(BE) = 1/2
(ED) = 5/2
.5 + 1.118 = 1.618
|
|
Extend arc (DG) through (A) to (I).
|
|
|
|
|
جواب |
رسائل 36 من 166 في الفقرة |
|
|
(BE) = 1/2
(ED) = 5/2
.5 + 1.118 = 1.618
|
|
Extend arc (DG) through (A) to (I).
Note the clear relationship between
phi and the square root of five.
|
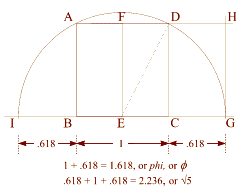
|
|
|
Solomons Temple also contains phi. The Vestibule (DCBA) measures twelve cubits by twenty cubits. 12 to 20 can be reduced to 6 to 10 and further to 3 to 5.
Three and five are two numbers in the fibonacci series. 3/5 = 1.6, a close approximation to 1.618, or phi.
|
|
Calendar I was measured very carefully by the NEARA/ASD Earth Mysteries Group in the early 80's.
Three measurements of the length were taken and averaged.
The same was done with the width. Upon dividing the length by the width, the resultant ratio was 1.619 to 1. Phi (Ø) = 1.618 to 1.
|

|
|

|
The Parthenon is the Queen of Greek Temples, and personifies their interest in Sacred Geometry. If the height of the Parthenon is 1, its width is phi (Ø) 1.618, and its length is root of 5= 2.236. And 1.618 + .618 = 2.236.
These are the 5 sacred geometrical ratios - Pi, (2),(3),(5) and Phi. They are found in sacred spaces all over the world. Remember, sacred geometry is basically simple. You must do it with your hands, if you want to really know sacred geometry.
|
Squaring the Circle - The Great Pyramid
|
The square represents the physical. The circle represents the spiritual. All sacred geometers have attempted the impossible: to square the circle (create a square who's perimeter is equal to the circumference of a circle.)
Here is the first of two valiant attempts:
This squaring of the circle works with a right triangle that represents the apothem (ZY) - (a line drawn from the base of the center of one of the sides to top of the pyramid), down to the center of the base (ZE), and out to the point where the apothem touches the Earth (EY).
|

The Great Pyramid of Egypt
(Sphinx in foreground)
|
|
|
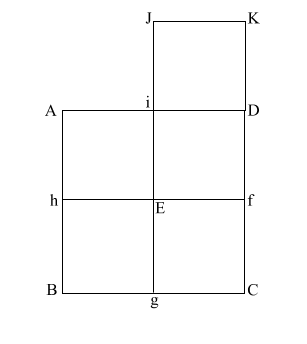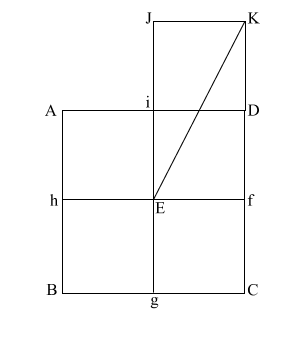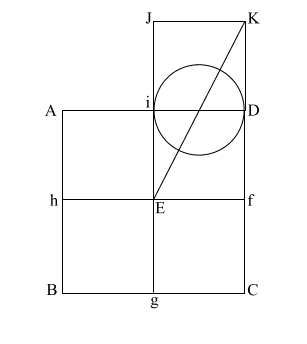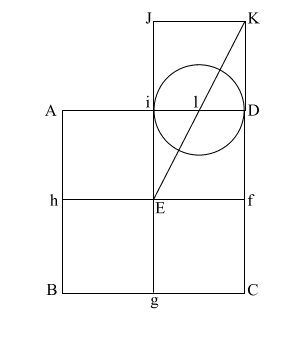
Now let's look at this in 2D,
from directly above.
For the purpose of this exercise,
the side (AB) of the base equals 2.
|
|

|
(ABCD) is the base of the Great Pyramid.
This is lettered similarly to the wire frame version (above).
For the purpose of this exercise,
the side (AB) of the base equals 2.
|
|

|
Construct square (i JKD),
thus creating double square (JKE f).
|
|
|
Create diagonal (EK)
which intersects (i D) at (l).
iD = 1,
therefore the diameter
of the circle is also 1.
(EK) = ( 5) = .618 + 1 + .618 5) = .618 + 1 + .618
|
|
|
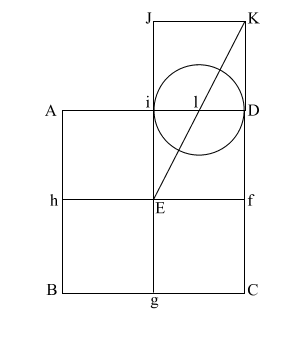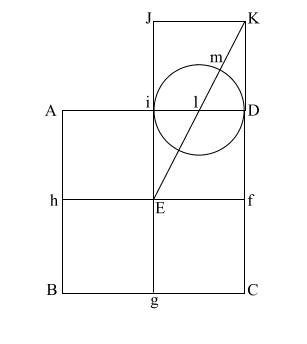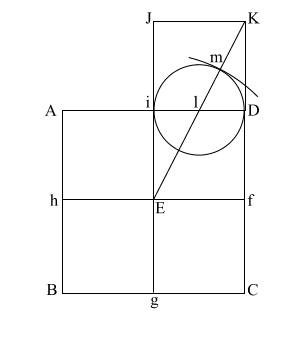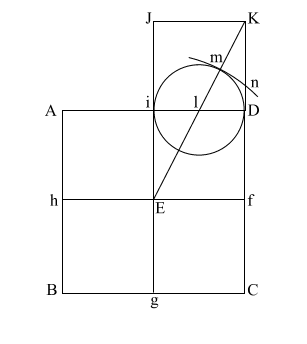
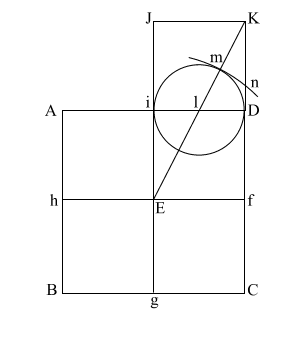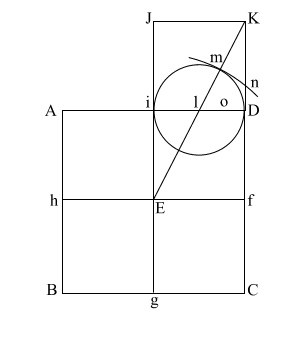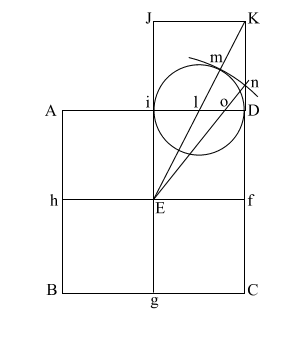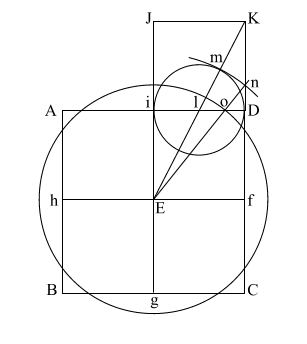
Put the point of your compass at (E) and extend it along the diagonal (EK) to point (m) where the circle intersects (EK), and draw the arc downward to intersect (KD f C) at (n).
If (EK) = ( 5), and (l m/l D) and l i = .5, the diameter of this circle is 1. 5), and (l m/l D) and l i = .5, the diameter of this circle is 1.
This makes (E m) = .618 + 1, or 1.618.
(E m) is the apothem.
|
|
|
Draw (E n) which intersects (A i l D ) at (o).
Put compass point at (f) and extend it to (n). Again put your point at (E) and draw the circle which happens to have the radius (E o).
(f n) is the height of the Great Pyramid.
This circle comes remarkably close to having the same circumference as the perimeter of the base (ABCD).
|
|
Let's go back to the original right triangle (EYZ)
(EY) = .5
(YZ) = phi
(EZ) = ( phi) phi)
EY = .5, The apothem is phi/1.618. This makes the 51 degree + degree angle.
Using a² + b² = c², this makes the height the square root of phi.
|
Squaring the Circle - The Earth & the Moon
|
Create a square (ABCD) with (AB) = 11
Create diagonals (AC) and (BD) crossing at center point (E)
Construct a circle which is tangent to square (ABCD) at f
|

|
|
Construct two 3 . 4 . 5 right triangles, with the 4 . 5 angles at (A) and (D).
Connect the 5 . 3 angles creating square (abcd) with side (ab) = 3
{4 + 3 + 4 = 11, or side (AD) of square (ABCD)}
Create diagonals (ac) and (bd) centering at (e)
Create a circle that is tangent to square (abcd) at four places.
|
|
|
Draw line (Ee) which intersects side (AD) at (F)
(EF) = the radius of the larger circle and (eF) = the radius of the smaller circle
The larger circle thus created is to the smaller circle as the moon is to the Earth!
With your compass point at (E), create a circle with radius (Ee)
This creates a circle whose circumference is equal to the perimeter of square (ABCD)!
|

|
The Math:
|
1
(AB) = 11
(EF) = 1/2 of (AB) = 5.5
(ab) = 3
(eF) = 1.5
Therefore 5.5 + 1.5 = 7
The circumference of a circle is equal to two times the radius (the diameter) times pi (3.1416).
C= 14 x 3.1416
C= 43.9824
|
2
In Square (ABCD), (AB) = 11
The perimeter of a square is four times one side. 11 x 4 = 44
According to the Cambridge Encyclopedia, the equator radius of the Earth is 3963 miles. The equator radius of the Moon is 1080.
The claim is that the smaller circle (in square abcd) is to the larger circle (in square ABCD) as the Moon is to the Earth.
|
|
3
(EF) = 5.5
(F e) = 1.5
5.5 : 1.5 :: 3963 : 1080
5.5 / 1.5 = 3.66666
3963 / 1080 = 3.6694 - (if it had been 3960, it would have been exact!)
|
Sacred Geometry
Geomancers are interested in sacred geometry because this is the study of the way that spirit integrates into matter - by echoing and amplifying the geometry of nature and planetary movements, we help to align the resonance of body/mind/spirit with the harmonic frequencies of the above and the below.
Geomancers are interested in sacred geometry because it has been found that certain spaces, with particular ratios, enable the participant to resonate or vibrate at the appropriate rate that maximizes the possibility of connection to the One.
A violin isn't built out of a cigar box! It is built with the proper wood with the proper shape and ratios, so that it resonates correctly for the notes/frequencies it is expected to produce. These same principles are applied to sacred spaces to maximize the possibility that whatever is being done there on spiritual levels will succeed.
Definitions
Two Dimensions
I've been a student of sacred geometry for over twenty-five years. While there has been recent interest in three-dimensional sacred geometry based on the Platonic Solids and in sacred sites themselves, most sacred geometrical documents I've read talk in only two dimensions - height and width.
Obviously there is a fourth dimension and others beyond it that are much more complex and sophisticated. But why does the record left to us from geomancers of the past come primarily in two dimensions?
Two is closer to the One than three is. It's less complex. I think one of the biggest mistakes Western geomancers have made was to take something that is very simple and make it much more complex. The Chartres Labyrinth strikes me as being an example of this. This stuff is simple. If you really gnow (that is, know both rationally and intuitively) a handful of irrational ratios - pi ( ), phi (Ø) and the square roots of two, three and five, you've basically got it all. ), phi (Ø) and the square roots of two, three and five, you've basically got it all.
Three-dimensional sacred geometry just builds on this basic handful.
Numbers
One aspect of Sacred Geometry is that it works with irrational numbers. To go to the spiritual, one must go beyond the rational, and it appears that some of these ratios and numbers can lead us there. By being inside a sacred space that has been constructed using one of a handful of these sacred geometrical ratios, the resonance that has been set up can enhance the possibility of your making the spiritual connection you want to make.
So, what are these irrational numbers? Let's begin with the rational.
Rational Numbers
A rational number is a number which can be expressed as the ratio of two integers (whole numbers), such as 1/3 or 37/22. All numbers which, when represented in decimal notation, either stop after a finite number of digits or fall into a repeating pattern, are rational numbers.
Irrational Numbers
An irrational number is one that cannot be represented as a ratio of any two whole-number integers, and consequently it does not fall into a repeating pattern of any sort when written in decimal notation.
All of the Sacred Geometry ratios we will be working with, the square roots of two (1.414), three (1.732) and five (2.238), phi (1.618) and pi (3.1416), are all irrational numbers.
Transcendental Numbers
There are certain kinds of irrational numbers that are called transcendental numbers. Just like irrational numbers, they are defined by what they are not (they aren't rational numbers), yet transcendental numbers are so identified because they are not another sort of number, known as an algebraic number.
Any number which is a solution to a polynomial equation is an algebraic number. A polynomial equation is a sum of one or more terms involving the same variable raised to various powers, for example:
7 (x5) + 5 (x3) + x = 137
Any X for which any such equation is true is an algebraic number. Because the square root of two is a solution to the polynomial equation,
x2 = 2
it is an algebraic number.
A transcendental number requires an infinite number of terms to be defined exactly. That's one way of thinking of God/dess. There are special equations to derive transcendental numbers where the terms get smaller and smaller as you go along, so you can keep adding them together to reach any level of accuracy you need, but the true number cannot be reached exactly. That is the beauty of transcendental numbers!
Pi ( = 3.1416...) is such a transcendental number. It is the only one we will be using here with Sacred Geometry. One infinite equation which relates to the value of pi ( = 3.1416...) is such a transcendental number. It is the only one we will be using here with Sacred Geometry. One infinite equation which relates to the value of pi ( ) is this: ) is this:
Pi / 4 = 1 - (1/3) + (1/5) - (1/7) + (1/9) - (1/11) + (1/13) - (1/15) + ...and so on into infinity.
http://www.bibliotecapleyades.net/geometria_sagrada/esp_geometria_sagrada_3.htm
|
|
|
|
جواب |
رسائل 37 من 166 في الفقرة |
|
|
|
|
جواب |
رسائل 38 من 166 في الفقرة |
|
http://josemanuelmatematicas.blogspot.com/2010/02/el-numero-de-oro-y-la-meca.html
http://josemanuelmatematicas.blogspot.com/2010/02/el-numero-de-oro-y-la-meca.html |
|
|
|
جواب |
رسائل 39 من 166 في الفقرة |
|
http://www.youtube.com/watch?v=Hip7pHZajI8
|
|
|
|
جواب |
رسائل 40 من 166 في الفقرة |
|
The Fibonacci Numbers:
Connections within the Mathematics and Calendrical Systems
of Ancient Mesoamerica
|
|
|
|
جواب |
رسائل 41 من 166 في الفقرة |
|
Daniel, the Magi and the Luni-solar Calendar of Israel
An interesting aspect of the teachings of the Magi comes from their founder, Zoroaster whose writings in the Zend Avesta, uphold much of the depth of meaning we found in Numbers 24:17. The Magi may have also applied this prophecy to the return of the older Zoroaster. The ancient roots of the Zoroastrianism lie in the ancient Persian traditions, and share many common elements with Judaism and Christianity. The tenets of the Christian Faith were preserved by God and handed down to other faithful men via the oral traditions, even as the truths of the Biblical Patriarchs were. The historical record bears this out, relating to the important point of how the Babylonian zodiac of 12 signs each of 30 degrees was transmitted to ancient Greece. Many scholars are not certain, as to the process by which this occurred, thus its an engaging point of history that both the ancient Greeks and Babylonians came under the rule of the Persian empire initiated by Cyrus the Great in 539 BC. It was during the rule of Darius I "the Mede" that the Prophet Daniel presided over his Court Astronomers and Chaldeans. Drawing on the rich astronomical traditions of the Biblical Patriarchs not to mention, the famous astronomical expertise of the Persian Magi, Daniel's potent influence convinced Darius to adopt some salient principles of Biblical Astronomy in the period around 522 BC. Certain important aspects of Zoroastrianism agreed with the Biblical standard practiced by the Hebrews, carried on by Daniel. The Magi would certainly have been familiar with Daniel's prophecy concerning the 70 weeks judged on Jerusalem relating to the coming Messiah, [Dan. 9:2, 24-27]. Daniel's influence had the added effect of preserving the astronomical truths of the promised seed, contained in the form of the zodiacal narrative, which God put in the most capable hands of the time. To illustrate this point more robustly, let's look at the practice of the Intercalation of months in the Hebrew Lunar Calendar, an important part of this practice of the Israelites in preserving their astronomical heritage.
We know the Hebrew practice of Intercalation of months dates back at least to the time of Moses, who instituted the sacred observances of the Old Testament Law during Israel's wanderings in the wilderness in the mid-second millennium BC. This preceded Meton of Athens who is given credit for discovering the saros in 432 BC, by at least a thousand years. The roots of the word "saros," and its practices however are much older. The Calendar of Israel is governed by the 354-355 day lunar year with twelve months of 29 or 30 days. The named months of the Israelite year coincide with the Babylonian months, including Adar Sheni, the extra 13th month which was added to keep the solar year aligned with the seasons. The practice of Intercalation of months kept the holidays of Passover, Pentecost and Rosh Hashanah, just prior to the Feast of Tabernacles in the month of Tishri, in their proper seasons of early Spring, the beginning of summer and at summer's end. The "11-day gap between the solar & lunar years expands to a period of seven months over this 19-year lunar cycle. In the Calendar of Israel a total of seven years (3rd 6th, 8th, 11th, 14th, 17th and 19th) during this 19-year lunar cycle, would normally contain an extra month of Adar Sheni."1
With every 19 solar years plus two hours, the Sun and Moon return to meet each other at the same appointed time and place in the sky. The saros, however, consists of 19 eclipse years, which is only 18 solar years plus eleven days. An eclipse year thus consists of 346.62 solar days, which is equal to 11.738 lunations. When we divide the saros by 11.738, we get 1.618, (or phi),2 accurate to 4 digits! Since the Sun intersects a lunar Node two times every 346.62-day eclipse year, we find the cycle of the celestial rendezvous of the Sun and Moon also governed by the phi ratio. The Hebrew calendar also unifies these luni-solar cycles according to phi, in concert with Israel's sacred fifty-year cycle of Jubilees. This is evident because there are 618 lunations in any given 50-year Jubilee cycle. Thus, as the Hebrew calendar shows the perfect correlation of the sacred and secular celestial cycles, governing the rhythms of a myriad terrestrial and cosmic cycles, we find more evidence of the signature of God the Creator and His rulership of light reflected in the heavens, the Source behind all kingdoms of earthly life. This exhibits the perfect relation between the civil [lunar], and sacred [solar] years of the Calendar of Israel, in the larger context of precession. These related standards of the sacred and secular are also unified in the promised seed, Jesus Christ as the Priest--King of Israel, and light of the world presiding over all circadian rhythms and other aspects of the kingdoms of terrestrial life.
As we briefly mentioned earlier, the roots of the word for saros are much older than Meton's application of the term. In fact it goes back at least as far as ancient Sumeria, because the Sumerian name for the great lunar eclipse cycle was also called the saros. This word traces through to the basic Babylonian unit of measure, also called a "sar," showing ancient elements of the sacred celestial rule of measure. This period of lunation consisting of 18 years & 11, (10.96) days, was utilized by the Chadean priests to predict eclipses. Wallis Budge supported this notion when he stated; "the Babylonians were a nation of stargazers observing the appearances of the moon, eclipses, & planets etc." This was the culture that produced the Magi, and speaks volumes on not only their astronomical expertise, but also their focus while watching the heavens for the celestial signs revealing the birth of the promised seed. The Chaldeans also had a period of 3600 (60 x 60) years that they called a saros. Here again we see the Sexegesimal pattern behind the organization of the heavens, including the cycle of the Moon joining Saturn and Jupiter every 60 years in their celestial dance. Jupiter-Saturn Conjunctions exhibit the essence of this "base 60" numerical system as part of its function as a cornerstone of Biblical cosmology, providing the context of the Celestial Prelude marking the birth of Christ. Additionally, it gives us a window on the "celestial mechanism" that frames all Creation, the precession of the equinoxes. This will be fully explained in the Specific signs of the Celestial Prelude. Both of these planets frame the ancient solar system as its two outer-most naked-eye planets, while opening a window on precession. It also shows a unity of Saturn's 29.5-year orbit with the 29.5-day lunar cycle on a year-day basis. This is half the Sexegesimal order of the solar system, formatted in the orbits of these two gas giants, the other half being Jupiter's near 12-year cycle spending a year in each zodiacal sign, even as the Sun spends a month in each of the 12 signs. The solar half of the order is based on a division of 60, by twelve: (12 x 5), and the lunar half by 30 [30 x 2], both multiples of sixty. This is part of the numerical order of the solar system as it was patterned by the Creator according to the phi ratio.
The Babylonian "Sar" is doubtlessly related to the Hebrew word [*H8269] of the same spelling, meaning; "prince, captain, chief, ruler, leader, head." The word has retained this meaning even in modern usage, as in the Tsar of all the Russias. As a testimony to what may have been based on the first human language, "Aesar" is a word for "God" in both, Icelandic and Irish, "Osar" in Egypt like "Osiris" was the prince. The English word "sir" and the Roman "Ceasars" are also traceable to this root. It is used in Daniel 10:13 & 20, referring the archangel Michael who dispatched the fallen angel (Daimon) who opposed the messenger angel that God sent to answer Daniel's prayer. In Isaiah 9:6, one of the great prophecies of the promised seed, it is used of the Messiah as Sar-Shalom; the Prince of Peace, the one who brings peace to all Creation [Eph. 2:11-18]. It is additionally used of Prince Moses in Exodus 2:16.
According to Hislop, The Chaldean version of this word; "Zer," meaning "to encompass" gives us not only the English basis for: "Zero signified by a circle among the Chaldeans," but also Zero; "the seed." Further, it relates to the Hebrew word zera [*H2233] used in reference to the promised seed in Genesis 3:15. To this end the Chaldean word for the "woman's promised seed" was "Zero-ashta," that also formed the basis of the name Zoroaster. We should note that in the writings of Zoroastrianism in the Zend-Avesta, the predicted return of Zoroaster as a savior who would renew all existence in preparation for the Last Judgment, was prophesied. These references to the woman's promised seed point directly to the general sign of the Celestial Prelude. Hislop also notes:
"In almost all nations, not only was a great god known under the name of Zero or Zer; the seed, and a great goddess under the name of Ashta or Ishta; the woman, but the great god Zero is frequently characterized by some epithet which implies that he is The only One." 3
This evidence provides a strong basis for a direct correlation to the decan Coma, the seed who was the desired of the nations, especially when we view the supernova in this child's head [pictured below from the Dendera zodiac] who was the promised seed, during the general sign of the Celestial Prelude. The implication that the promised seed of the woman would be the only one, speaks to the truth that Christ is the Alpha and Omega, or the first and last way God made available for the redemption of Mankind. Hislop goes on to say: "As he who by the Chaldeans was regarded as the great Seed was looked upon as the Sun incarnate, and as the emblem of the Sun was a circle, the hieroglyphic relation between zero; "the circle," and zero; "the seed" was easily established."4
The Biblical references to Christ associated with the Sun as the "light of the world," the "Sun of Righteousness" [Mal. 4:2] and in Psalm 19:4-6, are only a few of many such references. Thus, in the case of the promised seed, Zero-ashta, the religion of the Magi served as a vessel for many principles and astronomical precepts, that led Mankind to the realization of the promised seed.
Ignatius, one of the Church Fathers and the Bishop of Antioch, in about 69 AD, records that, "At the appearance of the Lord a star shone forth brighter than all other stars." If we are careful not to confuse this reference with the planet Jupiter, we can deduce that this supernova was still visible almost 200 years after it was first seen as the celestial marker of the general timeframe of Jesus' birth. Seiss has some viable historical references on this point:
"Hipparchus about one hundred twenty-five years before Christ, observed it [Coma supernova] as a new star, and was led by it to draw up his catalogue of stars. Ptolemy, about one hundred and fifty years after Christ, refers to it as having been observed by Hipparchus, but as having become so faint as hardly to be any longer discernible."5 (parenthesis and emphasis mine)
A point of interest here regarding Hipparchus is that he is generally credited with the discovery of the precession of the equinoxes, by modern science in about 134 BC. He reportedly came upon this discovery while studying the star Spica, on a celestial map produced by his predecessor, Timocharis. He noticed that Spica was about 2 degrees away from its location on the star map, and decided that it was moving an average of 1 degree every 75 years: [close to the actual 72 years]. This is a key to remember in our study of Dendera's zodiac related to Spica, and this general sign later in this study. Also during this same period referred to by Ptolemy above, shekels from Judea struck during the period of the second revolt, [132 AD.] bear the design of a bright star over the Jerusalem Temple, called Bar Kaukab, or Son of the Star. This would have also been a logical reference to the supernova that appeared in the head of the woman's son in Coma's lap, the desired of the nations, perhaps even a providential reference to Jesus as the true temple. The decan of Coma is pictured below as the three bright stars forming the inverted "L" shape, which significantly contains the North Galactic Pole, located 5 degrees west of Beta Coma. This is interesting in light of the original star picture associated with this decan, as seen in the Denderah zodiac, of a mother holding her Son in her lap, known as "the Desired of the Nations," [pictured above]. The close proximity of the Northern Galatic Pole, recalls the imagery of Cepheus "the crowned king" who regally sits astride the Celestial Pole star polaris, picturing Christ seated in the heavenlies at the right hand of the Heavenly Father [Eph. 1:20-23].
The Magi would have witnessed both this new star shining brightly in Coma, a decan of Virgo, while Jupiter was in retrograde, standing still on the Meridian in Virgo, in December of 2 BC. The general sign of our Celestial Prelude thus harmonizes with the specific signs, as the Magi headed south from Jerusalem, their visit with Herod just concluded, towards Bethlehem in search of the promised seed. This also explains why the Magi were the only group to recognize these specific celestial signs of the Messiah's birth, because the general sign was a fixture in Coma for about 200 years prior to this period. The typical person wouldn't have noticed anything out of the ordinary in the heavens, because it would not stand out unless coupled with the knowledge of the specific signs, which brought the Magi to Israel in the first place. This is another reason why the Magi were so joyful when they saw "his star," Jupiter over Bethlehem. This scenario of what the Magi beheld in the skies over Bethlehem in December of 2 BC, is depicted in the banner of this article above. In Numbers 24:17, when Jacob is referred to in Balaam's prophecy, it bears reference not only for the seed of Jacob, but also in the land promised to him as an inheritance, in his covenant with God [Gen. 15:18]. Rolleston has an interesting remark regarding this:
"The forewarned that the star must appear over Jacob, over his inheritance, would see that star in Coma passed over the center of that inheritance: but as it would also appear to pass vertically over every part of it that was nearly in the of Jerusalem, they could not at once fix on the spot of the Messiah's birth, therefore they went to inquire; the , as it were, being given by the star, the longitude by the prophecy.MagiLatitudeLatitude"6
Here we see the witness of the stars, and the Scriptures, working together to bring the to the toddler Jesus, because their alert observation of "his star" brought them to Jerusalem and the scribes declaration from Micah 5:2, when Herod questioned them, sent the to Bethlehem. While en route they witnessed the heavens come alive with the proof that their historic journey was not in vain, because they saw both the Coma , and Jupiter in Virgo in the same skies over Bethlehem.
In summary then, the key points regarding the general sign of the celestial prelude are first, that this in Coma, set the timeframe in which the Magi were to expect the specific signs surrounding the birth of Christ. Secondly, that when this general sign is separated from the set of specific signs of Jupiter-Saturn triple conjunctions, the confusion clouding the "Star of Bethlehem," for the most part dissolves.MagiMagisupernovasupernova
FOOTNOTES:
1 Symbols of Judaism M.A. Ouaknin p.12.
2 Sun, Moon and Earth. Robin Heath, p.28
3 The Two Babylons Hislop, p. 59
4 The Two Babylons Hislop, p. 18
5 Gospel in the Stars, Joseph Seiss. P.161.
6
http://www.try-god.com/view_page.asp?id=61
Mazzaroth; The Star of Bethlehem, Rolleston,
*Strong's Concordance word definition for H8269, H2233, etc.
|
|
|
|
جواب |
رسائل 42 من 166 في الفقرة |
|
|
|
|
جواب |
رسائل 43 من 166 في الفقرة |
|
|
Has publicado que a ti también te gusta esto. Deshacer
|
|
|
|
جواب |
رسائل 44 من 166 في الفقرة |
|
Here we have the dragon’s head associated with its tail, since the tail always does what the head tells it to do. In the graphic above from "Apian"s Astronomicum Caesareum," [Ingolstadt 1540 AD] depicting the alternating head & tail of the dragon, we find 27 [9x3] points on the circle around the central dragon. This relates directly to the lunar calendar, or the Draconic Month, also known in Astrology as the Head & Tail of the Dragon. As we stop to consider the geometry applied here, we find an interesting dynamic. This is a shadow of the pattern we saw above in the nine full series of colored curtains of 27 cubits in the Tabernacle, referring to the Sidereal Lunar Month of 27.322 days. Geometrically, the numbers 3:9:27 reflect a relationship where 9 is the geometric mean between 3 and 27. Thus, 9 equals the square root of 3 x 27, or 81. The Mayan knowledge of this is geometry is indicated in one of their most accurate lunar calendars, based on 81 full moons occurring every 2,392 days. Here we find a celestial image of the spiritual hierarchy of the enemy’s kingdom of 1/3 of the host of Heaven, based on an aspect of the lunar sidereal cycle, counted against the backdrop of the stars. We should also take note that the common ratio underlying all of this, without variation is 1:3. This invariant ratio, can also be expressed as 1/3, which reflects the third part of the angels dragged down to Earth in the dragon’s tail in Rev. 12:4 above. Thus we find common elements in the order of Cherubim depicted on the curtains of the Tabernacle, with the 27 days of the sidereal lunar month, as they correlate to the Draconic month of the dragon’s head and tail.
This ancient connection with dragon and the lunar cycle, is also witnessed in the fact that in Astrology, a lunar Node, the point where the Moon crosses over the ecliptic, was also called the dragon’s head and tail, dictating the periods of the eclipse cycle, according to the phi ratio. The Eclipse limits for both solar and lunar eclipses are dictated by a 6 degree range within 12.5° to 18.5° of the lunar Node, wherein a New Moon or Full Moon takes place. The accuracy of the Maya lunar calendar is indicated in its 11,960 days [2392 x 5] or 405 lunations, [260 x 46 in tzolkin terms] when divided [11,960÷ 405= 29.5308] gives us a lunar synodic cycle of 29.5308 days. When this lunar synodic cycle is divided by the 69 groups or families of days when solar eclipses occur, [29.5038÷ 69=173.333] we get the average period of 173.333 days between eclipses. As the Sun links with a lunar Node when two of these 173.333 periods elapse, it gives us an eclipse year of 346.62 days, 18.6 days short of the solar year of 365.242. This reveals the basis of the phi ratio because 18.618 x 18.618=346.62 telling us that the lunar cycle relates to the eclipse cycle via the phi ratio of 1.618.
This parallels the connection made by Richard H. Allen [p.249], that Hydra’s “winding course symbolized that of the Moon; hence the latter’s nodes are called the Dragon’s Head & Tail. When a comet was in them, poison was thought to be scattered by it over the world.”12
Since the six degree range of the lunar Node was formerly tied to Draco at the northern Celestial Pole, it indicates the time before the dragon was cast down to the earth with his spirit realm. However, after the dragon was cast down, its head and tail would be transferred from the polar regions, down to the ecliptic, where the Hydra is located. This powerful figure of casting down the dragon, with his 1/3 of the stars of heaven, [fallen angels] to the Earth with him, is symbolically depicted as a Meteor shower from the Constellation Draco, surrounding the period of Christ’s birth. For a deeper look into this topic, please review our website article: “Introduction to the Jupiter-Regulus Conjunctions.” Also noteworthy is the section on “Lunar eclipses and Occultations” under the study on the “Calendar of the Biblical Patriarchs.” This verse [Rev. 12:4] also relates directly back to the first prophecy of the promised seed in Gen. 3:15, where the seed of the woman would bruise the serpent’s head, while the serpent’s seed would bruise the man’s heel. As the dragon’s head directs the spirit realm in his tail, the desired acts of persecution and destruction against the woman’s seed are carried out by the serpent’s seed-those who follow the Way of Cain [Jude 1:11], in open rebellion against the true God. This reveals the overriding purpose of the serpent, devouring the promised seed of the woman, as soon as he was born. We saw an example of this in the actions of the serpent’s seed-King Herod, who murdered all the male children around Bethlehem under age 2, in an effort to devour the infant Jesus, as soon as he was born. Having failed at this, the enemy has turned his attention to the believers.This develops a fuller understanding of the link between the serpent’s seed and lunar symbolism, which we have referenced in other studies on this website.
(Rev 12:5) And2532 she brought forth5088 a man730 child,5207 who3739 was3195 to rule4165 all3956 nations1484 with1722 a rod4464 of iron:4603 and2532 her848 child5043 was caught up726 unto4314 God,2316 and2532 to his848 throne.
This verse encapsulates the victorious life, ministry & completed work of Jesus Christ, from birth to resurrection and beyond, in one verse of Scripture. This figure of speech is called symperasma, provides a summary and conclusion of the subject matter-the inevitable Coming and ultimate Victory of the Promised Seed. The emphasis here shows Christ’s ultimate victory over the serpent and its seed, despite all the enemy’s efforts to obstruct the purposes of the Heavenly Father. This emphasis is monumentally preserved in the Great Sphinx with the head of the Woman Virgo bearing the infant promised seed, who matures to fulfill his highest of calling, by defeating and destroying the enemy’s work, as shown in the other 10 zodiacal signs between Virgo and Leo. His final victory over the serpent is witnessed in Leo, the Lion of the tribe of Judah [Rev. 5:5], as the Lion’s extended claws are set to rend the Hydra to pieces. The Hydra is the longest of the Constellations, extending below Libra’s scales through Virgo and Leo to include the Crab of Cancer. These four signs; the woman, her seed, his sacrifice, and the altar, not only represent 1/3 of the entire circle of the 12 signs of the zodiac, but also reflect a carnal image of Christ’s righteous sacrifice, in the religion of human works, attempting to earn salvation while ignoring the completed work of Grace, given by God in His only begotten Son. Thus, as the Hydra [the serpent destroyed] slithers below these four signs of the zodiac, he represents the counterfeit and the deception of antichrist, leading away from, and denying the true work of Christ. This is an important aspect of the devil’s works that Christ came to destroy [John 10:10, I John 3:8].
Revelation 12:7-9
(Rev 12:7) And2532 there was1096 war4171 in1722 heaven:3772 Michael3413 and2532 his848 angels32 fought4170 against2596 the3588 dragon;1404 and2532 the3588 dragon1404 fought4170 and2532 his848 angels,32
(Rev 12:8) And2532 prevailed2480 not;3756 neither3777 was their848 place5117 found2147 any more2089 in1722 heaven.3772
(Rev 12:9) And2532 the3588 great3173 dragon1404 was cast out,906 that old744 serpent,3789 called2564 the Devil,1228 and2532 Satan,4567 which deceiveth4105 the3588 whole3650 world:3625 he was cast out906 into1519 the3588 earth,1093 and2532 his848 angels32 were cast out906 with3326 him.846
In verse 7 we have the Archangel Michael contending in spiritual warfare against the dragon and his angels even as we see below in Daniel 10. In verse 9 we find four names of the adversary [dragon, old serpent, the Devil, and Satan], referring to four different aspects of the enemy’s spiritual dominion over the darkness of this world. We also see a fifth name-deceiver, which is a common trait in the other four names of the enemy. The Devil must deceive people into following his ways, because nobody in their right mind would ever voluntarily follow him if they knew his true nature. Thus deception is a universal trait of the enemy’s kingdom, and his mode of operation. This shows how the enemy has marshaled his spiritual forces of fallen angels in direct Opposition to the Godly order, reflected both in the curtains of Moses’ Tabernacle, and in the symbols of the Cardinal Directions. We have just seentwo examples of how the serpentine aspects of the enemy’s spiritual dominion are celestially represented in the Dragon-Draco and the Hydra. We will now look at Serpens the serpent, followed later by Cetus the sea monster.

One aspect of Serpens that arrests our attention is the recurring theme of the dragon’s head and tail, which we discussed above in connection with the serpentine asterism of Draco. This alerts us to the idea that, as the 4D symbolism of the Cardinal Directions paints a picture of composite truth, so their shadows do also. Just as each of the enemy’s 4 names in Rev. 12:9, indicate different functions in how the adversary opposes God’s purposes, so these serpents in the sky indicate varying aspects of his activity, which when taken together, provide us a detailed picture of how he works his evil designs in his world kingdom. Therefore we should consider these four serpentine signs both individually and collectively, as shadows of the cardinal points, to glean the fuller lesson of their message. As Robert Burnham states:
”Serpens is the only star group completely divided into two portions: Serpens Caput [head] to the west of Ophiuchus, and Serpens Cauda [tail] to the east.”13
In support of this structure, the stars within this Constellation follow suit. Burnham points out that “the Beta star in Serpens marks the head… while the Theta star marks the end of the serpent’s tail.”14 [ppg.1764-65] He goes on to highlight an intriguing link to Roman myth, where “Serpens is a symbol of the divine healer Aesculapius who became the god of medicine, and who appears in the sky as Ophiuchus the serpent bearer.” 15 |
|
|
|
جواب |
رسائل 45 من 166 في الفقرة |
|
11 and Phi expansions
11 is the magical unseen number of the Lord, but in case you still can't see it and after reading the NUMBER 11 posting and can't understand how it relates directly back to Him and His Design, consider the additive series of 1 plus 5.
You might already know that the Fibornacci series of a multiplying population such as Adam and Eve started, approximates PHI with every new generation. Phi being 1.618, and hence every generation expands by 1.618 times its starting population size. With an additive series, we merely add the last number added to a sum with that sum to make a new sum... For instance 1+1= 2. The next number in the series being 2 plus the last number added which was 1, making the new sum = 3. Therefore the next Fibornacci number would be 3 plus 2 = 5. And then its 5 plus 3 making 8. The expansion of 5 to 8 at these first steps is starting to get to 1.618, as here it is 1.6 as 5 becoming 8 has expanded or been mulitplied by 1.6. Later in the expansion it gets right up to PHI itself of 1.618 ...... (SEE Phi Graphics)
The Fiboracci series then looks like this ......
1, 1, 2, 3, 5, 8, 13, 21, 34, 55, 89... etc. Can you see how it works now ? And how it relates to PHI.also called the Golden Section.
(And for future reference notice how the first 7 numbers add up to 33 1+1+2+3+5+8+13=33)
So let's make a series using 1 to 5 this time ....
1, 5, 6, 11, 17, 28, 45, 73, 118, 191, 309, 500, 809, 1309, 2118 ..... and then notice how 5 and 6 add up to 11, in the Graphics of the Mysteries of Templars below
And with 500 as a central point
191/500 = .382
309/500 = .618
500/500 = 1
809/500 = 1.618
1309/500= 2.618
and these ratios are a PHI expansion
.382 x (1.618) = .618
.618 x (1.618) = 1.000
1.00 x (1.618) = 1.618
etc......
So is 500 important in the Lord's Design, as a central focal point. Yes, the Lord's PHI Pyramid at Giza had a height of 500 feet. Its base of 792' including the wall, went up 500 feet the same as the height of Glastonbury Tor, which was used by Joseph of Armithia and Mary Magdalene and even King Arthur when he was there about 500 years later. Coincidence, again I would suggest, NO by DESIGN as even the Cydonian Mound used as a temple to the Lord of Lords was also 500 feet in height above the Maritian plain. ( SEE Spiral Pathway to Altar ) I mean even the Tabernacle of the Lord in His Temple of this whole Solar System has a height of 500 seconds, using time instead of feet as the unit of measure. (SEE Tabernacle of the Lord.) And so eventually we find out that whether in distance lengths or time, the Lord used the same co-related numbers, and they all go back to the magnifying power of PHI. Biblically the Lord used 50 years as a measure of time, 50 being a factor of 500 times 10. 50 years being called a jubilee, and 120 of them being the time frame of man's dominion over the Earth, the Time of the Gentiles...and this adds up to 6,000 Years, yielding Divisions of 1,000 years. So 50 and 500 are phi aligned to the Creator and His Creation, and even to our worship of Him.
But if you are hanging in there mathematically, let's go a step further, or should I see a major unit further, as the Lord in His Tabernacle has 1000 seconds for the orbit of His Heavenly Planet called the Earth. It takes a 1,000 seconds for us to revolve around His Sun/Son.....as Jesus is the center of the Universe. But the time involved in His Light reaching us is 500 seconds, as we are at a usual distance from Him of 93,000,000 miles and His light speed has been set at 186,000 miles per second. D/e = 930/1.86= 500 seconds.
But interestingly enough once again, we can visulaize mathematically this Astronimcal Unit (A.U.) of the distance of the Earth from the SUN because it is a standard measurement which shows phi relationships with the other planets. Similarly this A.U. distance is related magically and logically to the Absolute 33 factor or NUMBER as Batter found on his LIX website. And as you might have found out by now, 33 hertz is the very resonant frequency of quartz and phi pryamids. Are you catching the magnitude of the connections of the Lord ?
Batter even came up with this equation ... Golden Mean / 33 = A.U. Meaning that A.U. sacred distance is related directly to Phi and even to 33..... and hence we have further proof that the Lord created the distances and Magic ratios, according to His Design between the Spiritual and Physical realms. And it sure is comforting mentally, physically and spiritually to know He has everything under control. As everything works together for good, to THEM that love the Lord and know HIM as their Creator
In His Service
David
Confirmations, corrections, additions to
Davidjayjordan@yahoo.com
|
|
|
|
جواب |
رسائل 46 من 166 في الفقرة |
|
-
|
|
|
|
www.youtube.com/watch?v=f0f-LugkXts14 May 2009 - 10 min - Subido por kewserx
Number of Golden Ratio, mystery of Kaaba, Miracle of Islam and Koran, it is the high time for Divine Secrets ...
|
-
|
|
|
|
www.youtube.com/watch?v=hD2323zkQUM20 Abr 2009 - 10 min - Subido por HighFlyingDutchman
Presenting the clear scientific facts that the 'holy' city of Islam is in the Golden Ratio point of the world. It ...
|
-
Has publicado que a ti también te gusta esto. Deshacer
-
Has publicado que a ti también te gusta esto. Deshacer6 entradas - 5 autores - Última entrada: 29 Ago 2010
I've heard Muslims talk about sacred geometry and how Mecca is the Golden Ratio of the world, but it's all Greek (heh) to me. Could someone ...
-
Has publicado que a ti también te gusta esto. Deshacer
-
Has publicado que a ti también te gusta esto. Deshacer
-
Has publicado que a ti también te gusta esto. Deshacer
-
Has publicado que a ti también te gusta esto. Deshacer1 entrada - 1 autor - Última entrada: 31 Ene 2010
1.618~ (or its inverse 0.618~): Number of Golden Ratio is a mystery of ... The proportion of distance between Mecca and North Pole to the ...
-
Has publicado que a ti también te gusta esto. Deshacer
-
Has publicado que a ti también te gusta esto. Deshacer6 entradas - 4 autores - Última entrada: 21 Sep 2009
What do you make of this? can someone actually do the calculations and verify? Ahh... feels good to have an army of dissenters do the work for ...
|
|
|
 أول أول
 سابق
32 a 46 de 166
لاحق سابق
32 a 46 de 166
لاحق آخر
آخر
|