|
|
General: EL GRIAL, OSEA EL LINAJE, TAMBIEN SE TELETRANSPORTA Y SE TRASLADA EN EL TIEMPO
Scegli un’altra bacheca |
|
Rispondi |
Messaggio 1 di 109 di questo argomento |
|
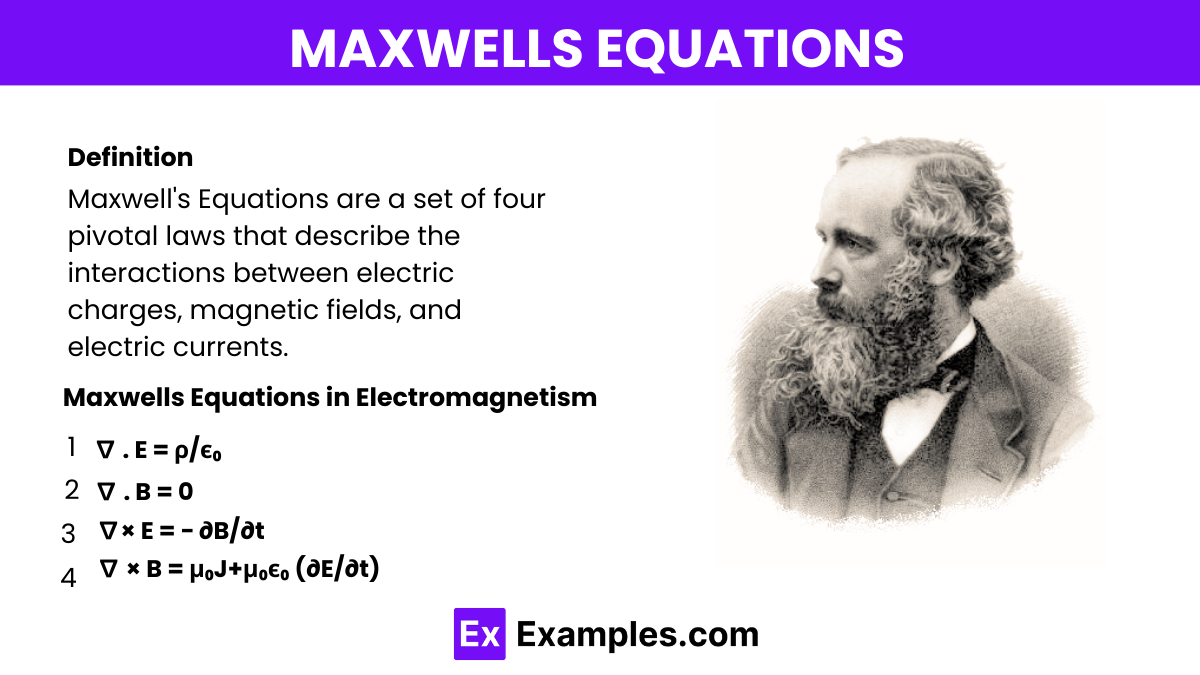
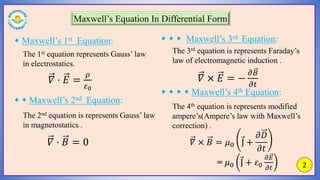
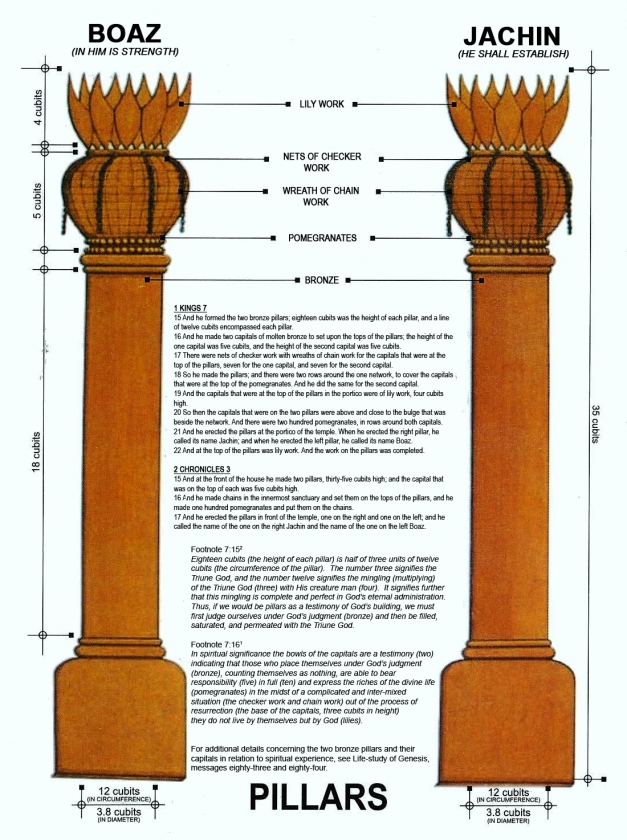
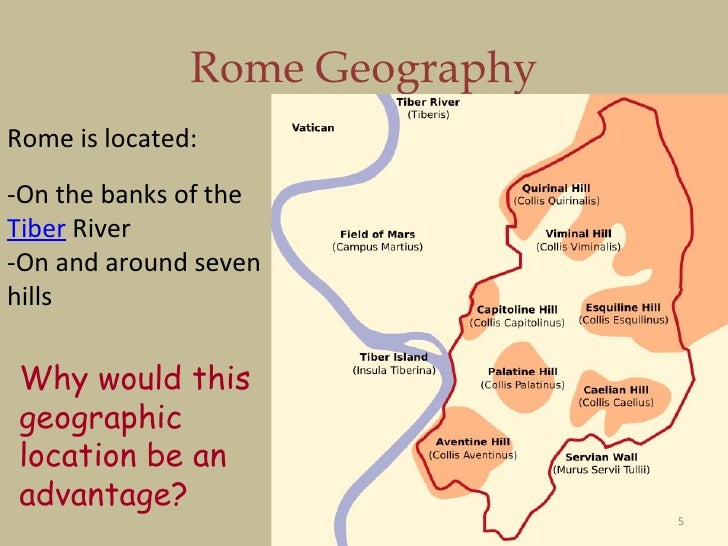
AQUI ESTA LA LLAVE DE LA ATLANTIDA. LAS DOS COLUMNAS DE JACHIN Y BOAZ, EN EL MARCO A LA PIRAMIDE. ES OBVIA LA REFERENCIA DE LA CREACION EN SEIS DIAS (TREINTA Y TRES).
PRIMER DIA=24 HORAS=12+12 HORAS
SEXTO DIAS=144 HORAS=12*12 HORAS
LA CLAVE DE LA SANTA CENA / "RELOJ DE LA CREACION DIVINA"
|
|
|
|
Rispondi |
Messaggio 95 di 109 di questo argomento |
|
agujero blanco=hombre=boaz
agujero negro=mujer=jachin
salmo 119 (alfa y el omega)
entre los mismos hay un obvio "agujero de gusano"
el hijo, es el OBVIO "AGUJERO DE GUSANO"
| Reply |
Message 281 of 281 on the subject |
|
|
|
|
|
|
|
|
|
Rispondi |
Messaggio 96 di 109 di questo argomento |
|
Génesis, 8
1. Acordóse Dios de Noé y de todos los animales y de los ganados que con él estaban en el arca. Dios hizo pasar un viento sobre la tierra y las aguas decrecieron.
2. Se cerraron las fuentes del abismo y las compuertas del cielo, y cesó la lluvia del cielo.
3. Poco a poco retrocedieron las aguas de sobre la tierra. Al cabo de 150 días, las aguas habían menguado,
4. y en el mes séptimo, el día dieciesiete del mes, varó el arca sobre los montes de Ararat. (EL ARCA DE NOE TAMBIEN ES UN "ARCA PERDIDA" Y EN ESTE MARCO TAMBIEN ES UN SIMBOLO DEL GRIAL POR LA FUERTE RELACION DEL CALENDARIO DE NOE CON LA GESTACION DE UN BEBE, OSEA 9 MESES LUNARES. SE REPITE ESTE PATRON DESDE EL 1 DE NISSAN HASTA EL 1 DEL DECIMO MES, OSEA 266 DIAS Y TAMBIEN DESDE EL 10 DE ISHAR HASTA EL 11/11, OSEA GENESIS 8:6, AL FINALIZAR LOS 40 DIAS. EL CONCEPTO DE UN MESIAS NO TIENE ORIGEN JUDIO, SINO QUE GENTIL. NOE NO FUE JUDIO. TODO EL NEXO ALQUIMICO EN EL MARCO AL MESIAS, OSEA UN NUEVO CESAR TIENE ESTA REFERENCIA EN EL MARCO A LA LUNA CRECIENTE.)
5. Las aguas siguieron menguando paulatinamente hasta el mes décimo, y el día primero del décimo mes asomaron las cumbres de los montes. (DESDE EL PRIMERO DEL PRIMER MES, OSEA ROSH HASHANAH HASTA EL PRIMERO DEL DECIMO MES, INSISTO, TENEMOS 266 DIAS=36 SABADOS LUNARES. ESTE ES EL OCTAVO DIA DE LA FESTIVIDAD DE JANUKAH)
6. Al cabo de cuarenta días, abrió Noé la ventana que había hecho en el arca, (ESTO ES DESPUES DE 40 DIAS DEL PRIMERO DEL DECIMO MES, LLEGAMOS AL 11/11 HEBREO, OSEA EL DIA NUMERO 306 DEL CALENDARIO LUNI-SOLAR HEBREO. DESDE EL 10 DE ISHAR O SEGUNDO MES HASTA EL 11/11 TAMBIEN TENEMOS 266 DIAS)
7. y soltó al cuervo, el cual estuvo saliendo y retornando hasta que se secaron las aguas sobre la tierra.
8. Después soltó a la paloma, para ver si habían menguado ya las aguas de la superficie terrestre. (LA PALOMA, OSEA UNA REFERENCIA A LA CONSTELACION DE COLUMBA, TIENE UN FUERTE NEXO ESOTERICO MARIA MAGDALENA EN EL CONTEXTO A LAS BODAS DE CANA EN UN MARCO CON EL SEPTIMO DIA. COLUMBA / COLUMBIA / COLOMBIA / WASHINGTON D.C / MARYLAND / VIRGINIA.)
9. La paloma, no hallando donde posar el pie, tornó donde él, al arca, porque aún había agua sobre la superficie de la tierra; y alargando él su mano, la asió y metióla consigo en el arca.
10. Aún esperó otros siete días y volvió a soltar la paloma fuera del arca.
11. La paloma vino al atardecer, y he aquí que traía en el pico un ramo verde de olivo, por donde conoció Noé que habián disminuido las aguas de encima de la tierra. (EL OLIVO TIENE FUERTE RELACION CON EL LINAJE, OSEA EL HIJO)
12. Aún esperó otros siete días y soltó la paloma, que ya no volvió donde él.
13. El año 601 de la vida de Noé, el día primero del primer mes, se secaron las aguas de encima de la tierra. Noé retiró la cubierta del arca, miró y he aquí que estaba seca la superficie del suelo.
14. En el segundo mes, el día veintisiete del mes, quedó seca la tierra.
15. Habló entonces Dios a Noé en estos términos:
16. «Sal del arca tú, y contigo tu mujer, tus hijos y las mujeres de tus hijos.
17. Saca contigo todos los animales de toda especie que te acompañan, aves, ganados y todas las sierpes que reptan sobre la tierra. Que pululen sobre la tierra y sean fecundos y se multipliquen sobre la tierra.»
18. Salió, pues, Noé, y con él sus hijos, su mujer y las mujeres de sus hijos.
19. Todos los animales, todos los ganados, todas las aves y todas las sierpes que reptan sobre la tierra salieron por familias del arca.
20. Noé construyó un altar a Yahveh, y tomanda de todos las animales puros y de todas las aves puras, ofreció holocaustos en el altar.
21. Al aspirar Yahveh el calmante aroma, dijo en su corazón: «Nunca más volveré al maldecir el suelo por causa del hombre, porque las trazas del corazón humano son malas desde su niñez, ni volveré a herir a todo ser viviente como lo he hecho.
22. «Mientras dure la tierra, sementera y siega, frío y calor, verano e invierno, día y noche, no cesarán.»

que.es
Volver a la página de fotos de
1352 × 2048 - 514k - jpg |

impactony.com
anexo a las Torres Gemelas
1024 × 768 - 106k - jpeg |

m80radio.com
Torres-Gemelas
970 × 546 - 241k - jpg |

impacto.mx
Las Torres Gemelas
900 × 500 - 117k - jpg |

inciclopedia.wikia.com
Archivo:Avion torres-gemelas.
500 × 625 - 53k - jpg |

taringa.net
Y sigue refiriéndose en la
672 × 542 - 59k - jpg |

taringa.net
bronx
726 × 1063 - 583k - jpg |

taringa.net
Conspiracion Torres Gemelas
1024 × 768 - 65k - jpg |
(. NOTEN LA CLAVE. ESTA ES LA TRADUCCION SEGUN LA BIBLIA DE JERUSALEN. C-SAR EN EL MARCO A LA LETRA C ES UNA REFERENCIA A LA LUNA CRECIENTE Y SAR ES PRINCIPE EN HEBREO SEGUN ISAIAS )9:6. ESTA PROMESA ES ETERNA. OSEA EL CONCEPTO DE UN MESIAS ES MIENTRAS DURE LA TIERRA. ESTA ESCRITO. HAY UN LINAJE SAGRADO DE JESUCRISTO Y MARIA LA MAGDALENA, CLARAMENTE EN FUNCION A GENESIS 8:4, EN EL MARCO A LA LUNA CRECIENTE, OSEA EL 17 DE TISHRI. TODO EL NEXO DEL C-SAR ROMANO, EN EL MARCO A LA CIUDAD DE 7 COLINAS, TIENE ESTE NEXO ESOTERICO. OSEA ES UN PUENTE CON EL "EXPERIMENTO FILADELFIA". WASHINGTON D.C TAMBIEN TIENE SIETE COLINAS. EL VERDADERO CALENDARIO LUNI-SOLAR ES CON LUNA NUEVA EN LUNA LLENA)
BENJAMIN / GUERRA / MARTE / SANGRE / LINAJE
BENJAMIN ERA UNA TRIBU GUERRERA SEGUN LA TORA. EN ESTE MARCO ES OBVIA LA REFERENCIA A MARTE / SANGRE / LINAJE
169. Romanos 11:1 Digo, pues: ¿Ha desechado Dios a su pueblo? En ninguna manera. Porque también yo soy israelita, de la descendencia de Abraham, de la tribu de Benjamín.
170. Filipenses 3:5 circuncidado al octavo día, del linaje de Israel, de la tribu de Benjamín, hebreo de hebreos; en cuanto a la ley, fariseo;
HECHOS 12:12 (MARCOS/ MARTE / MARZO/ PASCUA)
LLAVE DE ORO Y DE PLATA AL IGUAL QUE LA MANZANA
LAS MATEMATICAS SON EXACTAS.
GLORIA A DIOS TODOPODEROSO. QUE BELLAS SON LAS MATEMATICAS
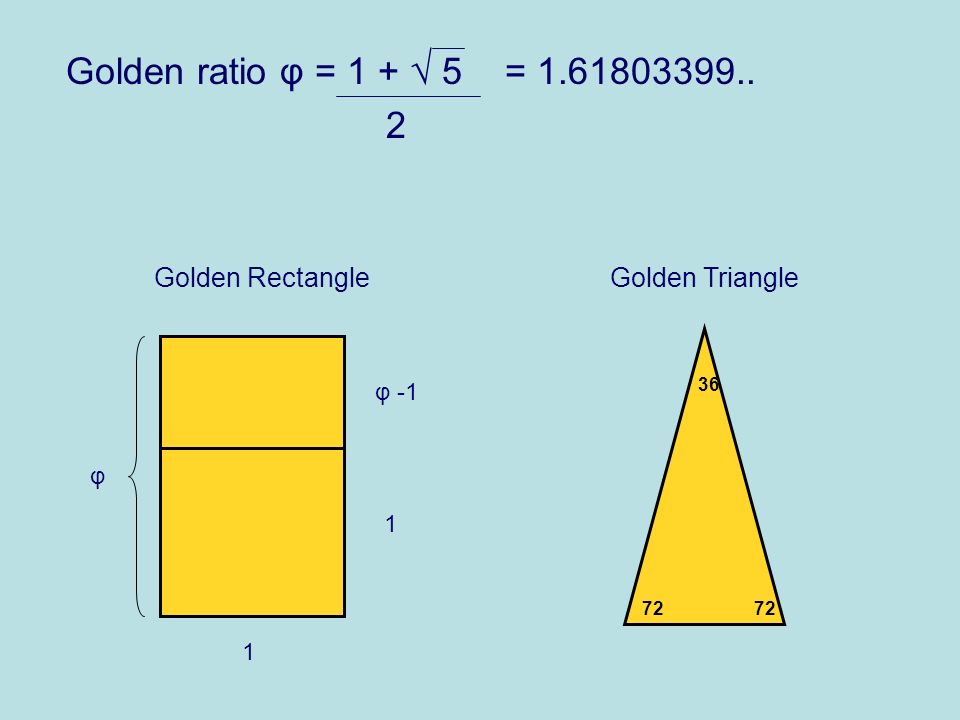
MARTE=MANZANA=1.618 =VATICANO= CASTILLO SAN ANGELO
La referencia a MARTE es un nexo con el tercer dia de la creacion (domingo=primer dia, lunes=segundo dia, marte=tercer dia), en el cual FUERON CREADOS EL MAR, LA TIERRA, LAS SEMILLAS, LOS ARBOLES Y LOS FRUTOS. En este marco EL ARBOL DE LA VIDA Y EL ARBOL DEL CONOCIMIENTO DEL BIEN Y EL MAL, tiene este nexo con el septimo via. Incluso en el marco a las BODAS DE CANA, que tambien tiene este mismo patron. ES SEPTIMO DIA en funcion a Juan 1:29,35 y 43 y tambien TERCER DIA en funcion a JUAN 2:1. ES OBVIO EL NEXO TERCER DIA EN EL MARCO AL SEPTIMO DIA. POR ESO EL NEXO MARTE CON EL GRIAL. BENJAMIN, AL HABER SIDO UNA TRIBU GUERRERA (GUERRA= SANGRE= ROJO= LINAJE= MARTE) TIENE ESTE NEXO.
En el libro de Juan (Yojanán), comenzando en Juan 1:19 hasta Juan 2:1 reseñaremos acontecimientos que sucedieron durante siete días de tiempo.
-
Juan 1:19 = Día Primero
-
Juan 1:29 = Día Segundo
-
Juan 1:35 = Día Tercero
-
Juan 1:43 = Día Cuarto
Después del cuarto día, el Mesías (Mashíaj) judío Yeshúa/Jesús marcha a Galilea. Galilea es la palabra 1551 de Strong en el Diccionario hebreo. La palabra Galilea en hebreo significa "un círculo." Un círculo es sinónimo del cielo. Estos cuatro días son un cuadro/anteproyecto espiritual dado a nosotros por el Di-s de Israel de que el Mesías (Mashíaj) judío Yeshúa/Jesús vendría a la tierra, después de haber estado en el cielo/círculo (Olam Habá) 4.000 años desde la creación de Adán en el Huerto del Edén (Gan Edén).
Juan 2:1 describe una boda en Caná de Galilea en el tercer día. Este tercer día (después de los cuatro días previos) es el séptimo día. En este día, hay una boda.
 Esto es un cuadro/anteproyecto dado a nosotros por el Di-s de Israel de que después de los 6.000 años de tiempo (el final del Olam Hazé), Él entrará en la plenitud del matrimonio con Su pueblo, cuando el Mesías (Mashíaj) judío Yeshúa/Jesús gobernará y reinará con Su Novia durante la Edad Mesiánica (Atid Lavó). En el pensamiento tradicional judío (casa de Judá), la Fiesta de las Trompetas (Rosh HaShaná) es el día de la plenitud del matrimonio del Di-s de Israel con Su pueblo. Esto es un cuadro/anteproyecto dado a nosotros por el Di-s de Israel de que después de los 6.000 años de tiempo (el final del Olam Hazé), Él entrará en la plenitud del matrimonio con Su pueblo, cuando el Mesías (Mashíaj) judío Yeshúa/Jesús gobernará y reinará con Su Novia durante la Edad Mesiánica (Atid Lavó). En el pensamiento tradicional judío (casa de Judá), la Fiesta de las Trompetas (Rosh HaShaná) es el día de la plenitud del matrimonio del Di-s de Israel con Su pueblo.
|
|
|
|
Rispondi |
Messaggio 97 di 109 di questo argomento |
|
53. Mateo 19:24: Otra vez os digo, que es más fácil pasar un CAMELLO por el ojo de una aguja, que entrar un rico en el reino de Dios.
54. Mateo 23:24: ¡Guías ciegos, que coláis el mosquito, y tragáis el CAMELLO!

  



53. Mateo 19:24: Otra vez os digo, que es más fácil pasar un CAMELLO por el ojo de una aguja, que entrar un rico en el reino de Dios.
54. Mateo 23:24: ¡Guías ciegos, que coláis el mosquito, y tragáis el CAMELLO!
JAVIER MILEI Y SU VISIÓN SOBRE EL RESENTIMIENTO DE LOS SOCIALISTAS
6 junio, 2017
El economista Javier Milei fue entrevistada por el diario La Nación en el marco de las entrevistas grabadas en video que lleva adelante el prestigioso matutino.
Dijo Milei “Fijate que en los últimos 40 años Argentina duplicó el tamaño del sector público con esta historia de la justicia social y la re distribución del ingreso”.
“La justicia social es una farsa. No hay nada más injusto que la justicia social, porque en realidad re distribuir el ingreso es un acto violento. Es un castigo al exitoso. Eso penaliza el crecimiento”.
“Suponete que yo tengo dos personas, una que básicamente no tiene más que segundo grado y un día estaba mirando a su mujer, y cuando la ve presta a ir a la cama se dice ‘pero yo tengo que inventar algo para que anden así las mujeres’, entonces inventa la bikini y se llena de plata”.
“Y al lado tenés otra persona que hizo su maestría, su doctorado, su post doctorado y se dedica a estudiar la patita trasera izquierda de las moscas”.
“Evidentemente el mercado ¿Que es lo que hace? El mercado premia a quien satisface mejor las necesidades de su prójimo. En un contexto que se relaciona con la escasez. Cuanto vos más exitoso sos en satisfacer las necesidades de tu prójimo, el mercado te premia y te llena de plata”.
“Entonces vos te encontrás con que el que inventó la bikini está lleno de plata y el otro que se mató estudiando está en una situación pobre, por decirlo de alguna manera”.
“Supongamos que el hombre de las bikinis tiene dos autos y el otro señor no tiene ninguno. Entonces se siente profundamente resentido porque mirá todo lo que estudió y no tiene auto y el otro tiene dos autos. Entonces ¿De que forma puede lograr para hacerse de un auto? La primera es buscar algo que satisfaga el mercado y de esa manera conseguir tener un auto”.
“Sin embargo hay otros mecanismos. Uno es ir y robarle una auto. Pero claro, eso es muy feo, ¿entonces que hace? Va y llama al matón del barrio para que lo intimide al hombre que inventó los bikinis para que le de un auto a él”.
“Entonces se siente tranquilo. La famosa re distribución del ingreso. La re distribución de la riqueza. Es decir un acto violento. Es una acto violento donde se castiga al exitoso y se premia el envidioso. Y cuando una economía entra en eso, que es básicamente la idea de la justicia social y el populismo te hundís. Y eso es lo que pasó en la economía argentina”.
“Vos tenés que se duplicó el tamaño del sector público en el PBI y el número de pobres se multiplicó por seis. Entonces cuando vos castigás al exitoso se castiga al proceso de acumulación de capital y ¿Sabés a quien termina perjudicando más? A los más vulnerables porque esos son los que no tienen capital. Y que necesitan del capital para ser más productivos y tener mejores salarios. Entonces la re distribución del ingreso lo único que hace es castigar a los más vulnerables”. Concluyó el economista Javier Milei.
http://infofarandula.com/javier-milei-socialistas/
EL RELATO BANANERO PARA LA "GILADA".
ENVIDIA, ENVIDIA, ENVIDIA O LA MISMA RELIGION DE JUDAS QUE QUIZO VENDER EL PERFUME MUY CARO, PARA DARLE A LOS POBRES, CON EL CUAL MAGDALENA UNGIO LOS PIES DE CRISTO EN BETANIA.
EL DIEZMO BIBLICO EN LA BIBLIA NO ES PROGRESIVO, OSEA QUE EL QUE DESDE EL QUE TIENE CIEN MIL MILLONES DE DOLARES HASTA EL POBRE, PAGA EL 10%. CONCRETAMENTE EL TODOPODEROSO NO ESTIMULO EL SOCIALISMO PROGRESIVO (EL QUE MAS TIENE MAS PAGA). OBVIAMENTE QUE UNO TIENE QUE AYUDAR AL POBRE, PERO ES UNO, NO EL ESTADO, PORQUE GENERA EL POPULISMO Y EL FEUDALISMO. EL QUE TIENE MAS, POR MEDIO DE LA OFRENDA, PUEDE AYUDAR OBVIAMENTE CON MAS DINERO, PERO PRETENDER DEMONIZAR AL RICO, EN FUNCION A LOS POBRES, ES POPULISMO BANANERO QUE TIENE ORIGEN EN LA ENVIDIA.
TODOS LOS VIVOS SON SOCIALISTAS CON LA PLATA DE OTRO.
¿CASUALIDAD O CAUSALIDAD?
|
|
|
|
Rispondi |
Messaggio 98 di 109 di questo argomento |
|
|
|
|
Rispondi |
Messaggio 99 di 109 di questo argomento |
|
|
|
|
Rispondi |
Messaggio 100 di 109 di questo argomento |
|
 


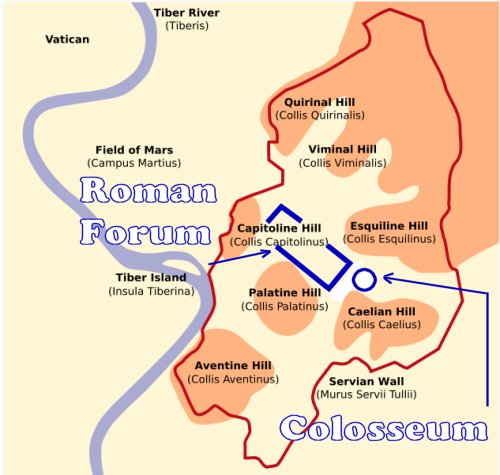
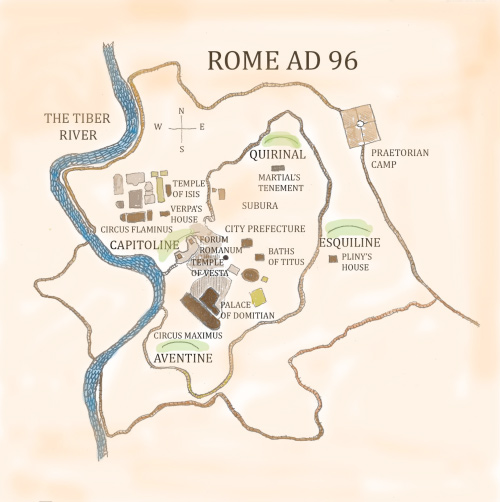
11 DE SEPTIEMBRE O SEPTIEMBRE 11
11/9 O 9/11
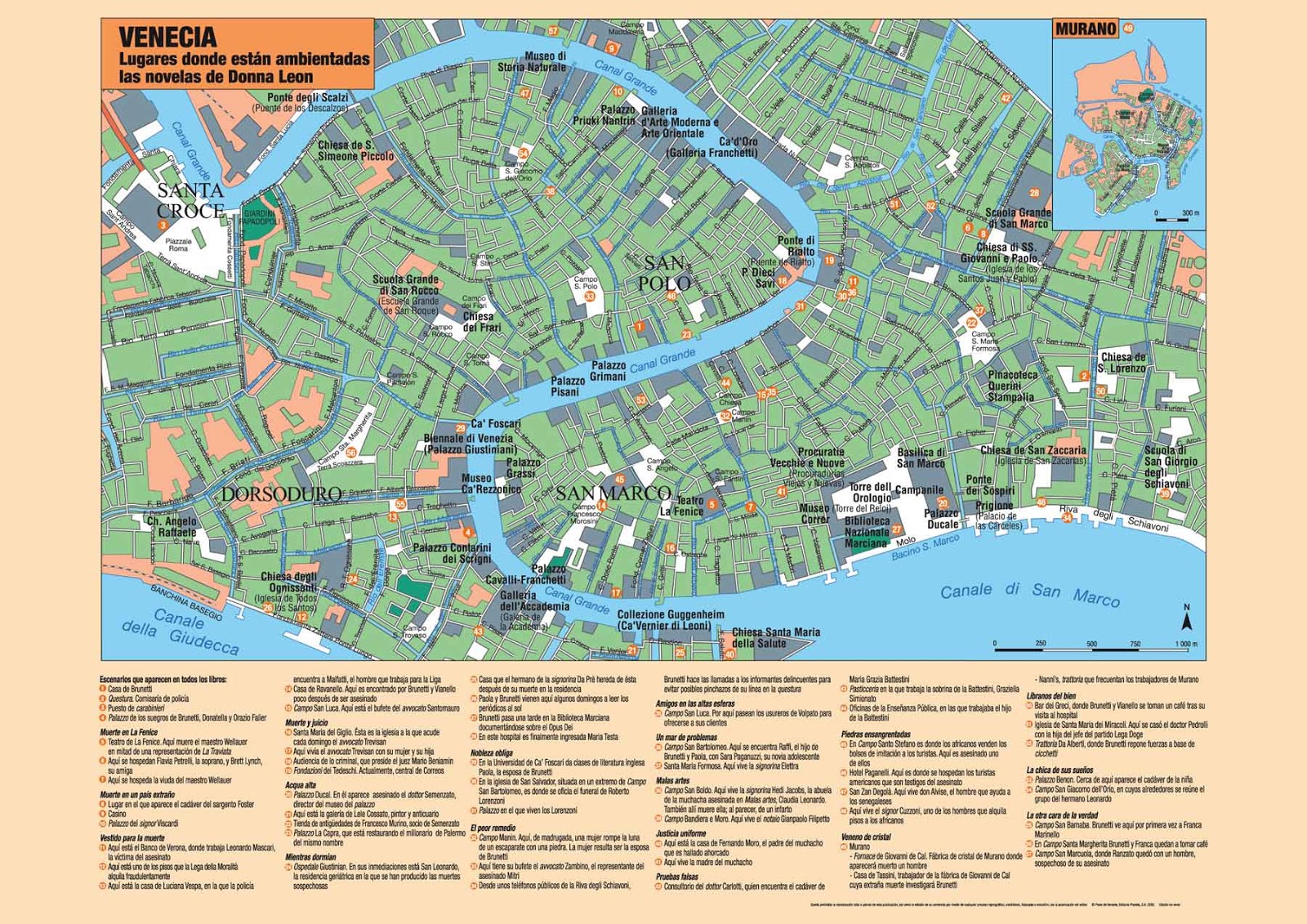
Sapientia Aedificavit Sibi Domum. Es decir, "la sabiduría ha edificado aquí su casa". Resulta curioso que la misma frase aparece en el Evangelio de María Magdalena, un texto apócrifo. Se dice que en el interior de esta iglesia y de otras muchas de Venecia está escondido el tesoro de los templarios. Pero no hay ninguna prueba de ello. Para terminar ya con esta entrada me gustaría que nos acercásemos un momento a uno de los edificios más emblemáticos de Venecia: el Palacio Ducal.

La Maddalena

La Maddalena

Tomb of Tommaso Temanza
 
ISLA SAN GIORGIO (VENECIA)=GEORGE LEMAITRE
GEMATRIA EN INGLES DE SEED=33
GEMATRIA EN INGLES DE GATE=33
SARA (CE-SAREA DE FILIPO)=PARALELO 33
"¡Oh profundidad de las riquezas de la sabiduría (sophia)
y de la ciencia (gnwsiV, gnosis) de Dios!
¡Cuán incomprensibles son sus juicios, e inescrutables sus caminos!"
(Romanos, 11: 33).
25 DE ABRIL=DIA DE SAN MARCOS
22 DE JULIO=DIA DE MARIA LA MAGDALENA
 the Apple
| milky way in Simple Gematria Equals: 119 |
( |
m 13 |
i9 |
l 12 |
k 11 |
y 25 |
0 |
w 23 |
a1 |
y 25 |
) |
| queen mary in Simple Gematria Equals: 119 |
( |
q 17 |
u 21 |
e5 |
e5 |
n 14 |
0 |
m 13 |
a1 |
r 18 |
y 25 |
|
| hebrew calendar in Simple Gematria Equals: 119 |
( |
h8 |
e5 |
b2 |
r 18 |
e5 |
w 23 |
0 |
c3 |
a1 |
l 12 |
e5 |
n 14 |
d4 |
a1 |
r 18 |
| mary magdalene in Simple Gematria Equals: 119 |
( |
m 13 |
a1 |
r 18 |
y 25 |
0 |
m 13 |
a1 |
g7 |
d4 |
a1 |
l 12 |
e5 |
n 14 |
e5 |
|
|
|
|
|
Rispondi |
Messaggio 101 di 109 di questo argomento |
|
Llevamos años soñando con el teletransporte. Y aunque ha sido una idea sugestiva y apasionante en la ciencia ficción, nunca ha dejado de ser una quimera. Pero poco a poco, en las últimas décadas, avanzado poco a poco en nuestro conocimiento del mundo y, sobre todo, en nuestras teorías de cómo lograr que el teletransporte sea una realidad.
En los últimos años, incluso hemos conseguido hacer pequeños experimentos y teletransportar fotones a más de cien kilómetros de distancia.Por eso, nos hemos preguntado en qué estado estaba todo esto y, sobre todo, cuánto falta para poder decir aquello de "Teletranspórtame, Scotty".
¿Tiene sentido hablar de teletransporte o, en realidad, no hace falta?
En 2013, se movieron 9.600 millones de toneladas por mar, unas 10.000 millones de toneladas en tren y sólo en España se movieron más de 178 millones de toneladas por carretera. De Shangai a Valencia, un barco carguero invierte casi un mes de viaje. A poco que hagamos cuentas: veremos que la cantidad agregada de tiempo que invertimos en mover cacharros por el mundo es una barbaridad.
Pero no seamos materialistas: Los belgas pierden 51 horas al año en atascos. Los holandeses, 41 y los alemanes, 39. En España, se pierden 24 horas por persona y solo en México DF se pierden más de tres millones de horas al día. Renfe mueve unos 500 millones de pasajeros cada año frente a unos 10 millones del transporte marítimo nacional.
Podríamos seguir, pero creo que se entiende la idea: el transporte es uno de los grandes cuellos de botella actuales. Coches autónomos, cohetes reutilizables y el famoso hyperloop, demuestran que es un sector en plena efervescencia. Pero ¿y si pudiéramos jubilar todo esto?
¿Qué entendemos por teletransporte?
Poca gente sabe que ni en Star Trek veían plausible el tema del teletransporte. La idea inicial era mostrar el despegue y el aterrizaje del Enterprise en cada planeta, pero el gasto en efectos especiales era tan grande que se tuvo que descartar. El plan b fue, sencillamente, no salir nunca de la nave. Pero, como era previsible, la serie se convertía en algo un poco aburrido.
Ahí fue cuando a Gene Roddenberry, el creador de la serie, decidió rescatar la idea de la teletransportación de La Mosca una película de 1958. Desde ese momento, Star Trek trabajó duro para convertir el teletransporte en el método de transporte más seguro de la galaxia. Vale que de vez en cuando combinaba personas (como en La Mosca) o las duplicaba, pero en general miles de personas lo usaban todos los días sin mayor problema.
De hecho, el mayor problema es que, bueno, es ciencia ficción. A día de hoy no es que no estemos cerca de conseguirlo es que ni siquiera tenemos claro que queramos hacerlo. Por lo que sabemos hasta ahora, solo hay una cosa mayor que los problemas técnicos que presenta el teletransporte: los filosóficos. Pero vaya paso a paso.
El teletransporte cuántico
En 1993, un grupo de físicos teorizó la única tecnología de teletransporte a la que tenemos acceso: la teletransportación cuántica. Ésta permite la transmisión del estado cuántico de unas partículas determinadas a otras partículas diferentes que se encuentran a una cierta distancia.
Es decir, se transmite la información, pero no la materia: simplificando un poco, la teletransportación cuántica no sería capaz de mandar cada átomo de nuestro cuerpo a otro lugar. Lo que podemos mandar mediante este sistema es el estado cuántico; es decir, son los planos exactos de nuestro cuerpo. De esta forma, los átomos de los que está compuesto un ser humano tendrían que estar en el lugar de llegada y con esta tecnología, los ordenaríamos para generar el cuerpo original.
A la decepción inicial, hay que sumarle que tampoco es instantáneo. El protocolo del teletransporte cuántico requiere enviar dos bits clásicos por cada cubit (la forma en la que 'nos encontramos' la información del estado cuántico que queremos transmitir). Para teletransportar dos cubits hacen falta cuatro bits y así sucesivamente hasta juntarnos con un conjunto inmenso de información. Da igual donde estemos: para transportar el estado cuántico de una persona se necesita tal cantidad de información que tardaríamos más en mandarla que en transportar a la persona físicamente. Las cuentas son sencillas: en el libro “La física de Star Trek”, Lawrence Krauss calculaba que la información del estado, las coordenadas y los niveles de energía que están ocupando los electrones de cada átomo pesa alrededor de un kilobyte. Si el ser humano está compuesto por 6,7 1027 átomos, es evidente que la cantidad de información que hay que mover no se puede transmitir ni en unos segundos, ni en varios millones de años.
Debido a todo esto, muchos han pensando que, en realidad, de lo que estamos hablando es de un lento sistema de clonación. Algo así (y perdonad el spoiler) como la máquina de El truco final (el prestigio). Pero también tengo malas noticias aquí. En 1982, otro grupo de físicos desarrollaron el teorema de no clonación. Se dieron cuenta de que es imposible crear una copia idéntica de un estado cuántico (esos planos de los que hablábamos antes) sin destruir el original. O sea, que para teletransportarnos tienen que matarnos y despiezarnos hasta dejarnos en los átomos. Por eso, la conclusión a la que terminamos llegando es que la máquina de teletransporte es una 'cabina de suicidios'
¿Qué somos? ¿Qué queremos ser?
Cuando hablamos de "identidad personal" en este ámbito no hablamos de como reconocemos o identificamos a la gente. Lo que tratamos de hacer es identificar qué necesitan alguien en un momento A y otro en un momento B para que sean la misma persona. Si nos fijáramos solo en la apariencia, por ejemplo, bastaría con un poco (o un mucho) de cirugía estética para engañarnos. Y todos estaremos de acuerdo que por cambiar el aspecto físico de una persona no se convierte, automáticamente, en otra persona.
A poco que empecemos a pensar posibles respuestas a la pregunta de la sección, veremos que es tremendamente difícil. Podemos decir que es tener el mismo cuerpo (pero constantemente estamos perdiendo y ganando células) o tener la misma personalidad o recuerdos (pero nosotros mismos no tenemos exactamente la misma personalidad o recuerdos que hace años).
La tentación es recurrir al concepto de 'mente'. Pero también tiene un problema y gordo: no tenemos ni idea de qué es. Aunque hay científicos que están explorando hasta qué punto nuestro cerebro podría sobrevivir al teletransporte, la verdad es que ni siquiera sabemos si la 'mente' es un fenómeno cuántico. Es decir, no sabemos siquiera si el cuerpo que se desintegra en el principio podría transferir su 'conciencia' al cuerpo resultante al final del proceso.
Entonces, ¿En qué ha quedado el teletransporte?
En poco, la verdad. Hemos podido hacer algunos experimentos y teletransportar algunas cosas diminutas. Pero, por ahora, es totalmente inviable teletransportar cosas más grandes. No sólo no podemos hacerlo, es que no tenemos ni idea de cómo hacerlo. Así que pese a las noticias que aparecen todos los años, el teletransporte no deja de ser algo muy exótico que es útil para conocer mejor la peculiaridades del mundo cuántico y para mantener entretenidos a los filósofos (y a los que nos interesan estos temas).
https://www.xataka.com/investigacion/tiene-sentido-todo-eso-del-teletransporte |
|
|
|
Rispondi |
Messaggio 102 di 109 di questo argomento |
|
|
|
|
Rispondi |
Messaggio 103 di 109 di questo argomento |
|
Científicos de Austria y China han logrado por primera vez teletransportar estados cuánticos tridimensionales. Esto podría desempeñar un papel importante en los futuros ordenadores cuánticos.
Investigadores de Austria y de China han demostrado experimentalmente lo que antes era solo una posibilidad teórica. Han logrado teletransportar complejos estados cuánticos de alta dimensión.
En su estudio, los investigadores teleportaron el estado cuántico de un fotón a otro distante. La información cuántica que se transmite se llama cúbit y es el análogo cuántico del bit. Es decir, un cúbit, como un bit, contiene la información de dos estados distintos, por ejemplo con valores '0' o '1'.
Sin embargo, los científicos lograron teletransportar un estado de tres niveles, el llamado cútrit. En la física cuántica es posible que '0', '1' sean ambos simultáneamente o, incluso, algo intermedio, a diferencia de la informática clásica. El equipo ha demostrado en la práctica una tercera posibilidad: '2'.
Cómo se hace la teleportación cuántica compleja
Desde los años 90 se sabe que la teletransportación cuántica multidimensional es teóricamente posible. Sin embargo, los científicos tuvieron que diseñar un método experimental para realizar este experimento.
El estado cuántico que será teleportado se codifica en los posibles caminos que puede tomar un fotón. Se puede imaginar estos caminos como tres fibras ópticas. En la física cuántica un solo fotón también puede ser localizado en las tres fibras ópticas al mismo tiempo.
Para teletransportar este estado cuántico tridimensional, los investigadores usaron el concepto de los estados de Bell. El método se basa en un divisor de haz multipuerto, que dirige los fotones a través de varias entradas y salidas y conecta todas las fibras ópticas entre sí. A través de una selección de ciertos patrones de interferencia, la información cuántica puede ser transferida a otro fotón lejos del fotón de entrada, sin que los dos interactúen físicamente.
El concepto experimental no se limita a tres dimensiones, sino que puede extenderse a cualquier número de dimensiones, subraya Manuel Erhard, uno de los autores del estudio.
Aplicación práctica
El experimento siginifca un paso importante hacia el futuro internet cuántico, ya que los sistemas cuánticos de alta dimensión pueden transportar mayores cantidades de información que los cúbits.
"Este resultado podría ayudar a conectar los ordenadores cuánticos con capacidades de información más allá de los cúbits", dijo Anton Zeilinger, físico cuántico de la Academia Austriaca de Ciencias y de la Universidad de Viena, sobre el potencial innovador del nuevo método.
Los investigadores chinos también ven grandes oportunidades en la teletransportación cuántica multidimensional. "El cimiento de los sistemas de redes cuánticas de próxima generación se basa en nuestra investigación fundacional actual", afirmó Jian-Wei Pan, de la Universidad de Ciencia y Tecnología de China.
El estudio fue publicado en la revista Physical Review Letters.
https://mundo.sputniknews.com/ciencia/201908241088480112-cientificos-logran-por-primera-vez-realizar-una-teletransportacion-cuantica-compleja/ |
|
|
|
Rispondi |
Messaggio 104 di 109 di questo argomento |
|
22/7=DIA DE MARIA LA MAGDALENA EN LA IGLESIA CATOLICA
BABILONIA LA RAMERA ES UNA MUJER Y ES LA MISMA BESTIA
BABILONIA LA RAMERA ES UNA MUJER Y ES LA MISMA BESTIA
PARA NO CREER PERO ESTO ES ASI. LA RELACION DEL DINERO / MONEY / MOON / CON LA LUNA EN EL CONTEXTO A LA TRANSFIGURACION EN EL MONTE HERMON / MON / LUNA EN LA TRIBU DE DAN / DINERO / SERPIENTE. TODO ES UN NEXO CON LA TRANSFIGURACION DEL SEÑOR. DINERO ES TIEMPO MISMO.
ES OBVIO EL NEXO DE LA SERPIENTE CON LA MUJER
NOTEN EL NEXO DE DAN, CON LA SERPIENTE (VENECIA) Y EL CABALLO (PLAZA SAN MARCOS)
7. Génesis 49:17 Será Dan SERPIENTE junto al camino, Víbora junto a la senda, Que muerde los talones del caballo, Y hace caer hacia atrás al jinete.
 1200 1200

|
|
|
|
Rispondi |
Messaggio 105 di 109 di questo argomento |
|
|
|
|
Rispondi |
Messaggio 106 di 109 di questo argomento |
|
|
|
|
Rispondi |
Messaggio 107 di 109 di questo argomento |
|
|
|
|
Rispondi |
Messaggio 108 di 109 di questo argomento |
|
|
|
|
Rispondi |
Messaggio 109 di 109 di questo argomento |
|
|
|
 Primo Primo
 Precedente
95 a 109 de 109
Successivo Precedente
95 a 109 de 109
Successivo
 Ultimo
Ultimo
|
|
| |
|
|
©2025 - Gabitos - Tutti i diritti riservati | |
|
|




















































































































































































/s3.amazonaws.com/arc-wordpress-client-uploads/infobae-wp/wp-content/uploads/2019/02/22181319/Sophie1.jpg)