One of our readers, John Frewen-Lord, speculates that the metre may be the modern version of a measure that was familiar to the Pharaohs.
While we think of today’s metric system (SI) as mostly a modern invention (1960), we have been led to believe for many years now that its most fundamental base unit, the metre, originated in France in 1793, and represented one ten-millionth of the earth’s quadrant (the distance from the earth’s equator to the North Pole, as measured at sea level) . Yet just a few years ago, the late Pat Naughtin discovered that the proposal for a universal standard of length very close to the metre may in fact have originated much earlier, via Bishop John Wilkins, an English cleric and philosopher, and a member of the Royal Society, in the mid-1600s. Recent comments on Metric Views now bring even that assertion into doubt, with the discovery of a measuring device called the wand having been around much longer still.
It is known that the wand, divided into ten segments, was almost exactly, to within a few millimetres, the same length as today’s metre, and that it was used as long as 1000 years ago. But what if all these versions of the metre were simply the rediscovery (or the handing down over time) of a standard measure, equating to the metre, that was invented in Egypt over 4500 years ago?
When we think of units of measure used in Biblical times, the cubit usually springs to mind. In fact, opponents of metric conversion have often referred to the cubit, in jest at least, as having as much validity as the metre. Such people should be careful for what they wish for, for, as we shall see, the cubit and the metre may in fact be directly related – and remarkably both are directly traceable to the Great Pyramid at Giza.
At first sight, such direct relationship may not be immediately apparent. There are a number of variations of the cubit, each different in length, but it is accepted that the Egyptian royal cubit is the definitive cubit, of which a physical example is on display in the Liverpool museum. Used to set out the Great Pyramid, its length measures 524 mm, or 0.524 m. For anyone hoping to see a nice round relationship between the cubit and the metre, I’m afraid the story is much more complicated than that! But keep in mind that number of 0.524 – for it will crop up again.
Let us look briefly at some of the mathematical properties of the Great Pyramid. Apart from the fact that it is just 3/60ths of a degree off an orientation of true north (the Prime Meridian through Greenwich is 9/60ths of a degree out of such an alignment), the Great Pyramid contains some quite stunning dimensional relationships between the numerical constants of pi (?), phi (?) and Phi (?) – and those relationships involve a dimension that is exactly equal to today’s metre. Let us explore this a bit further.
We all know what pi is. It is the ratio of a circle’s circumference to its diameter, and is approximately equal to 3.1416 (another number to keep in mind). We are probably less familiar with Phi and phi. One is the reciprocal of the other, with values of 1.618 and 0.618 respectively. The value of 1.618 is known as Phi with a capital P (?), while the reciprocal 0.618 value is represented by the lower case phi (?), and the two collectively are known by many names, such as the Golden Ratio, the Golden Mean, the Golden Number, and others, but they are values that exist throughout nature. Their discovery is attributed to mathematician Fibonacci in the 13th century.
Fibonacci noted that much of nature – and indeed much of Roman architecture – encompassed relationships of 1.618 and 0.618 for various aspects of design, and that these relationships relate to what is known as the Fibonacci sequence, consisting of 0, 1, 1, 2, 3, 5, 8, 13, 21, 34, 55, 89, 144, and so on, where each number is the sum of the previous two numbers. What is not always realised is that if you take any two adjacent numbers, say 55 and 89, you can obtain two ratios – 1.618 if you divide the second by the first, and 0.618 if you divide the first by the second (the minor variations in the decimal places get smaller as the numbers get bigger, coinciding at infinity). The Golden Ratio has a few unique properties – in fact these equations work only with the Golden Ratio and nothing else:
? = 1 + ? (i.e. 1.618 = 1 + 0.618);
? = 1/? (i.e. 1.618 = 1 ÷ 0.618);
? + 1 = ?² (i.e. 1.618 + 1 = 1.618² = 2.618);
? – 1 = 1/? (i.e. 1.618 – 1 = 1 ÷ 1.618 =0.618).
If we skip alternate numbers in the Fibonacci sequence, we end up with the same result as either ?² or adding 1 to ? – e.g. 144 ÷ 55 = 2.618 = 1.618 + 1 = 1.618² (keep in mind also the number of 2.618). Now you may be saying that this is all very interesting, but what has it got to do with the Great Pyramid at Giza, let alone the origins of the metre? All will become clear!
It is well known and accepted that the Great Pyramid incorporates the value of ? in its geometry – this was discovered by Englishman John Taylor in 1859, when he found that if you divide half the length of the Pyramid’s base perimeter by its height, you end up with ?. The base length of one side is 230.3 m, while its original height is 146.6 m. Therefore (230.3 x 2) ÷ 146.6 = 3.1418 – not precisely ?, but then the height of 146.6 m is at best an estimate of just how high the Pyramid was 4500 years ago (the very top is now missing, as is part of its external cladding, and ground level has likely changed). Likewise, take a circle with the same circumference as the perimeter of the base of the Great Pyramid. Calculate the radius of this circle. It will be found to be exactly equal to the Great Pyramid’s height (230.3 x 4 = 921.2. 921.2 ÷ (2 x 3.1416) = 146.6).
We must note that these relationships, along with many other relationships embodied in the Great Pyramid, can be made using any measurement units – they are not exclusive to the metre.
The Golden Ratio ? is there as well. If we take the surface area of the four sides, and divide that by the area of the base, we come to the value of ? (4 x 0.5 x 230.3 x 186.4 ÷ 230.3² = 1.618). Again, that is purely a ratio, and is not dependent upon any particular unit of measure. But now let us do some more calculations involving the Great Pyramid’s geometry that are dependent upon the metre – and only the metre.
- If we add two of the sides of the Pyramid’s base together, then subtract the height, we end up with a rounded value of 100 x ? (230.3 x 2 – 146.6 = 314.0).
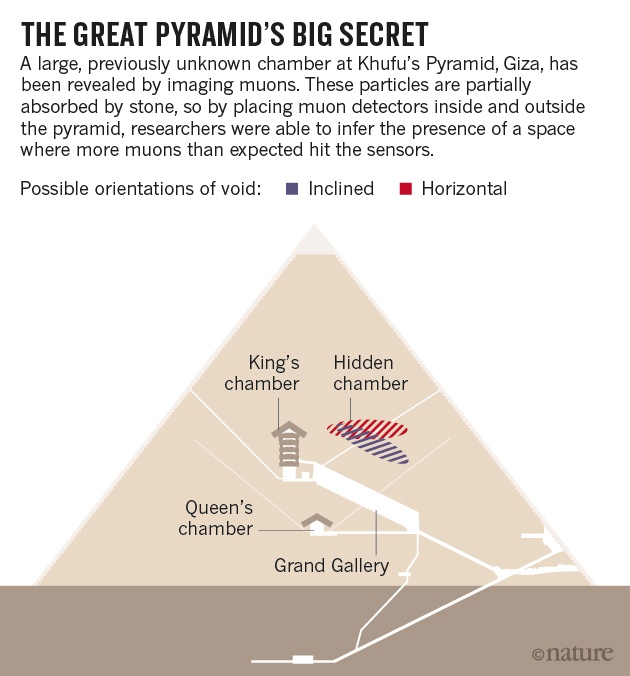
- The King’s Chamber measures 5.24 m x 10.47 m. The Chamber’s perimeter = 10 x ? (31.42 m). There are also many measurements in the King’s Chamber that relate to even multiples of ?, but only using metres.
- If we draw two circles, one circumscribing the Pyramid’s base (i.e. intersecting the four corners) and one inside (i.e. touching the mid-point of each side), then subtract, in metres, the circumference of the inner circle from that of the outer circle, you end up with a figure of 299.71. This is almost exactly one millionth of the speed of light in metres per second (299 792 458 m/s – the slight discrepancy is due to rounding at various points along the way).
Hold on – the ancient Egyptians may have known about the metre, but surely they didn’t know about the second? Perhaps they did. The length of two sides of the base of the Great Pyramid is the distance a point on the equator moves through space in exactly one second.
I’m sure if you tried hard enough, the Great Pyramid may be found to contain some mathematics that support imperial measures, even though things like the foot and inch were not anywhere near close to existence 4500 years ago, and anyway are promoted as being based on human properties, not mathematical ones. But there is one thing that really does indicate that the ancient Egyptians were very familiar with the metre. I mentioned early on in this article that the cubit, which was used to build the Great Pyramid (each side has a length of 440 cubits), was 0.524 m long, an apparently odd relationship to the metre. Let us however look at three equations:
- One sixth of ? is 0.5236 – to all intents and purposes exactly the length of the cubit in metres (to within 0.4 mm of the known physical example, and even that assumes that this example’s stated length has not been rounded to three decimal places); quite why one sixth is not clear, but the Great Pyramid is located exactly 30° above the equator – i.e. one sixth of the distance between the two poles.
- One fifth of ?² (2.618) = 0.5236 – again, exactly the length of the cubit in metres. There are five increments of 72° in a circle of 360°. It is known that the earth wobbles slightly on its axis, at the rate of 1° every 72 years.
- ? – ?² (3.1416 – 2.618) = 0.5236 – another relationship that yields the length of the cubit in metres, and ties together, by means of the cubit (and hence the metre), the two constants that are embedded in the Great Pyramid’s mathematical properties.
These equations cannot be pure chance or coincidence, but must have been created by a society that knew all about the metre 4500 years ago, and from which they derived the cubit. One thing is certain – no measurement unit can be more natural than the metre, based as it is on nature’s constants of ? and ? (not to mention the circumference of the earth). Clever people, those ancient Egyptians.
[Note: I claim little original material in this article, but have made extensive use of sources from Wikipedia, YouTube and others, all of which must be treated with the usual caution as to their absolute accuracy. J F-L]
https://metricviews.uk/2013/06/07/was-the-metre-invented-by-the-ancient-egyptians-4500-years-ago/











































![SOLVED: Learning Task 1: Complete the table by converting the measured distance of planets into the scale cm [0 million km]. Write your answer on your answer sheet. Use the following formula](https://cdn.numerade.com/project-universal/previews/599b118c-ce80-4be3-9973-a1175cc3be85.gif)










